Logistic growth
| Topics: |
Logistic growth population models imply density dependent population regulation. Such a model assumes that when populations increase in size (1) the per capita birth rate decreases (as a result of competition for resources) and/or (2) the per capita death rate increases (as a result of competition for resources, predation, or the increased spread of disease). Thus, there is a population size at which the per capita birth rate equals the per capita death rate. At this population size, known as the carrying capacity, the population growth rate is equal to zero.
Contents
Logistic growth equation
 The relationship between per capita birthrate (b, green line), per capita death rate (d, blue line) and population size in populations growing logistically. (Source: Phil Ganter, Modeling Density-Dependent Population Growth)
The relationship between per capita birthrate (b, green line), per capita death rate (d, blue line) and population size in populations growing logistically. (Source: Phil Ganter, Modeling Density-Dependent Population Growth) The logistic growth equation can be written as
dN/dt = rmaxN((K - N)/(K)) or dN/dt = rmaxN(1 - (N/K))
where dN/dt is the population growth rate, rmax is the maximum value that the per capita growth rate can be for a particular species in a particular environment, K is the carrying capacity, and N is the population size.
Thus, in logistic growth, the per capita growth rate decreases as population sizes increase.
r = rmax ((K-N)/K)
If N < k then r > 0, if N = k then r = 0, and if N > k then r < 0. Thus, populations increase in size when the population is smaller than the carrying capacity, decrease in size when populations are larger than the carrying capacity, and do not change in size when the population is at the carrying capacity.
Patterns of logistic population growth
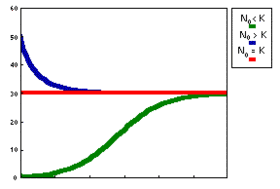 The plot of how population size varies over time when (1) the initial population size is much smaller than the carrying capacity (green line), (2) the initial population size is much large than the carrying capacity (blue line), and (3) the initial population size is at the carrying capacity (red line). (Source: Alexeii Sharov, Virginia Tech University)
The plot of how population size varies over time when (1) the initial population size is much smaller than the carrying capacity (green line), (2) the initial population size is much large than the carrying capacity (blue line), and (3) the initial population size is at the carrying capacity (red line). (Source: Alexeii Sharov, Virginia Tech University) Logistic growth can be visualized in a graph plotting how the population size varies over time. How population size changes over time in logistic growth depends on the initial population size.
Initial population size smaller than the carrying capacity (N<
When the initial population size is much smaller than the carrying capacity, the resulting graph is known as the "s curve". In logistic growth (1) over time the population size increases until the population size equals the carrying capacity and then the population size remains at the carrying capacity and (2) populations initially grow slowly and over time the population growth rate increases until it reaches a maximum (at N = 1/2k). As time goes on the population growth rate declines to zero where it remains.
Initial population size much larger than the carrying capacity (N>>k)
When the initial population size is much larger than the carrying capacity (1) the population size decreases until it reaches the carrying capacity and (2) strongly negative growth rate slows over time and becomes less negative until the growth rate reaches zero.
Initial population is at the carrying capacity (N = k)
if the population is initially at the carrying capacity, then the population growth rate will be zero so the population size will not change.
History of logistic growth model
Logistic model was developed by Belgian mathematician Pierre Verhulst (1838) who suggested that the rate of population increase may be limited, i.e., it may depend on population density.
Further Reading
- Campbell, N.A., J.B. Reece, and L.G. Mitchhell. 2006. Biology. Addison Wesley Longman, Inc. Menlo Park, CA. ISBN: 080537146X.
- Raven, P.H., G.B. Johnson, J.B. Losos, K.A. Mason, and S.R. Singer. 2008. Biology, 8th edition. McGraw Hill, New York, NY. ISBN: 0073227390.
