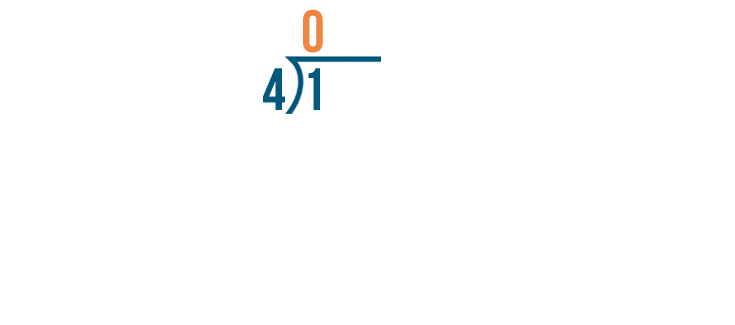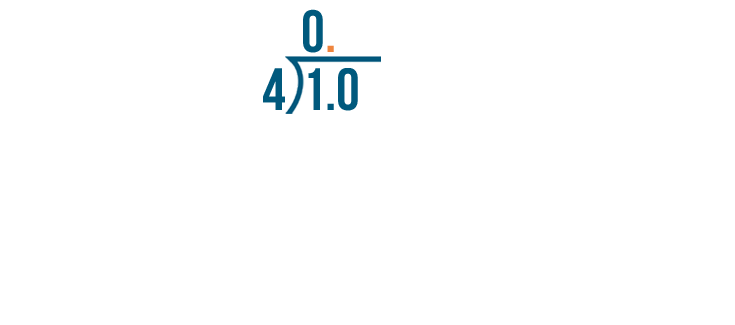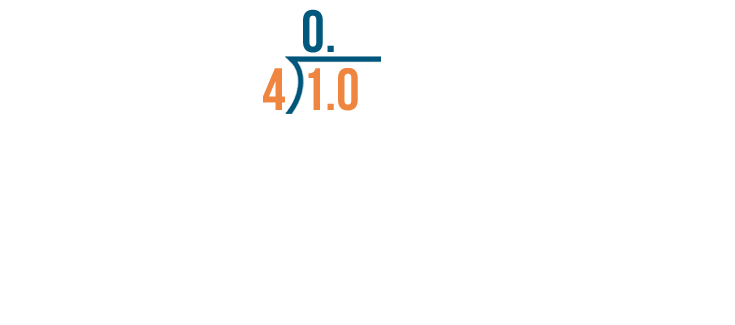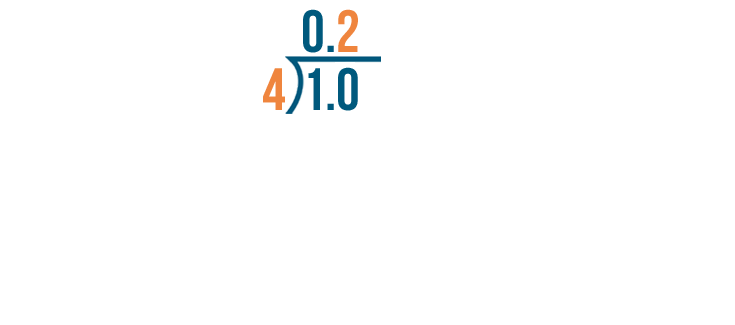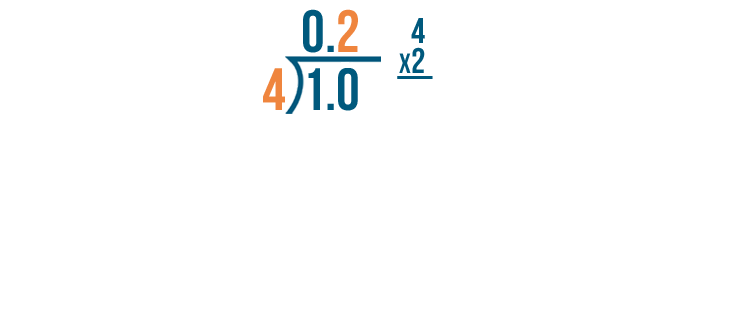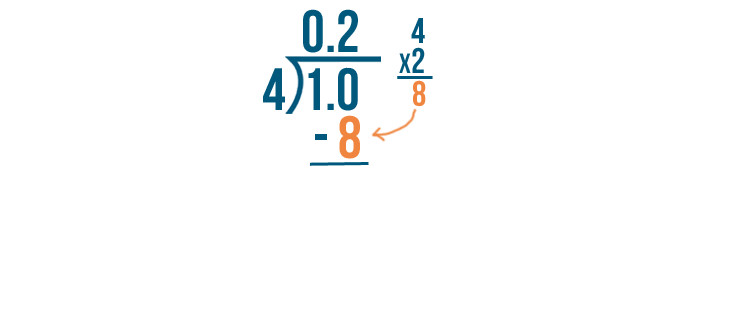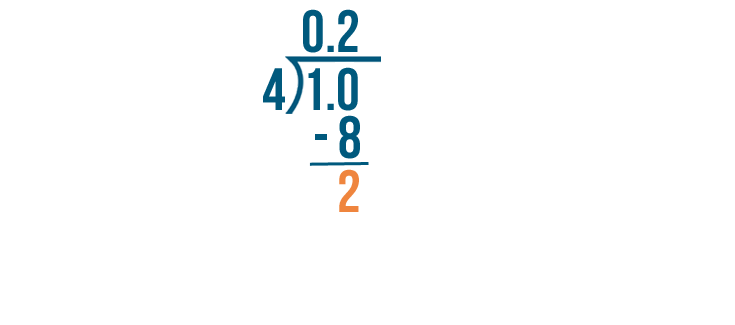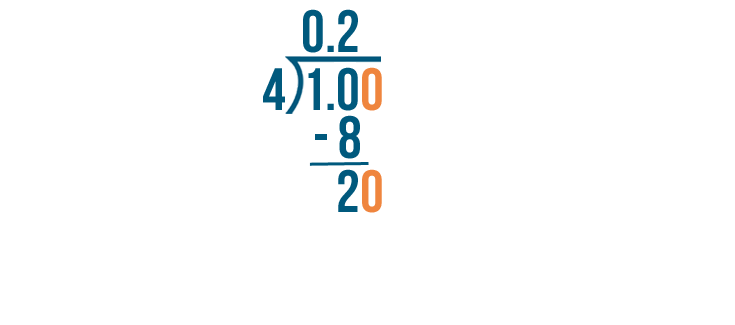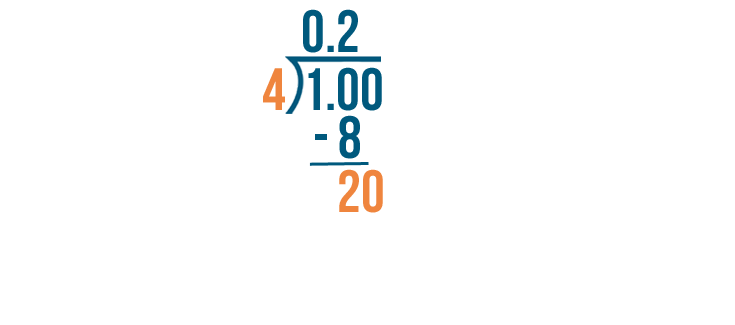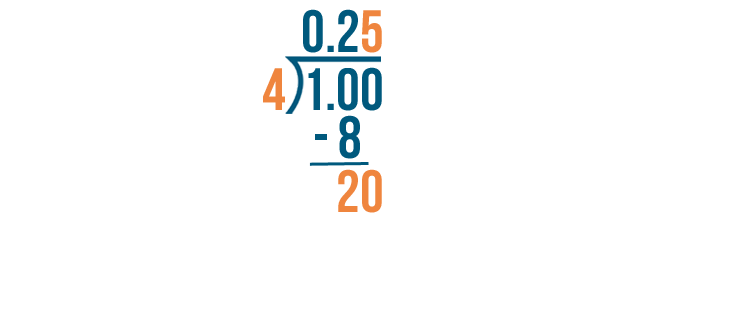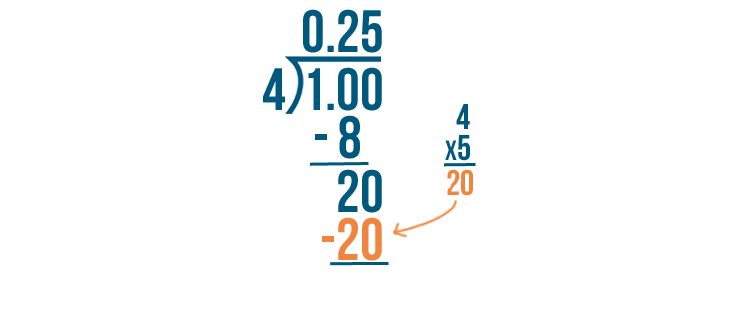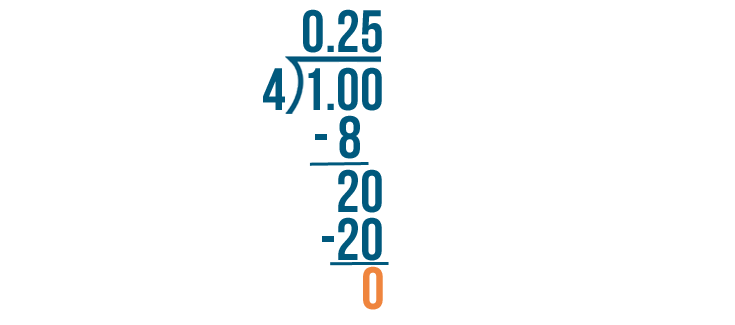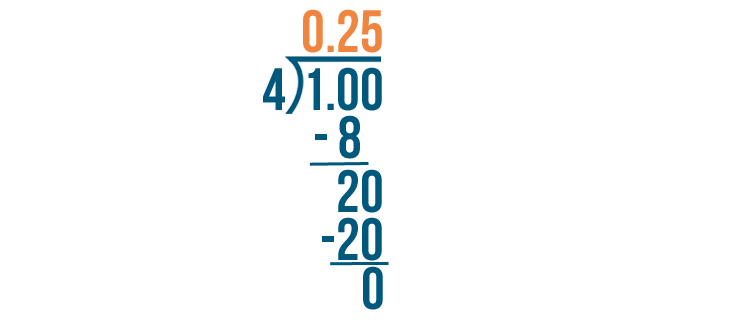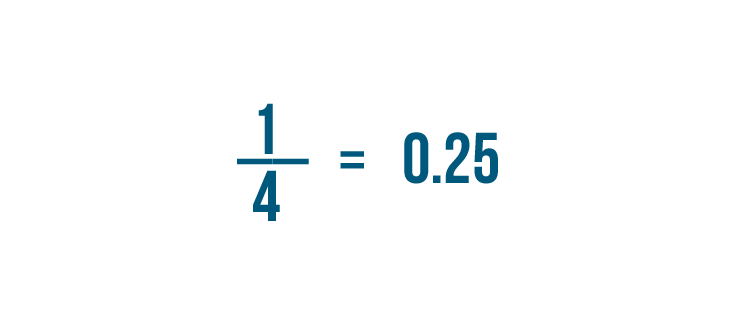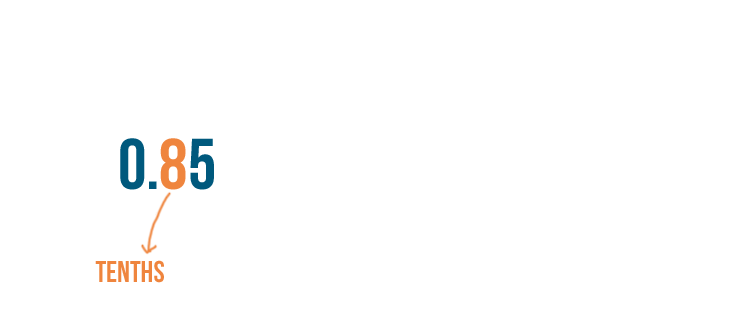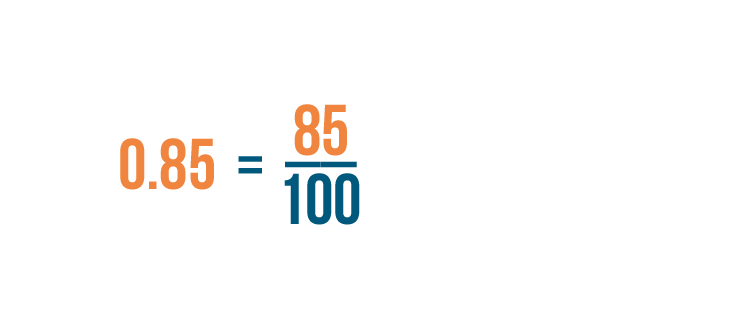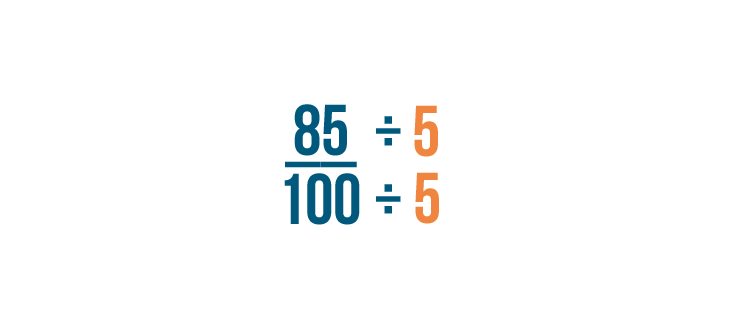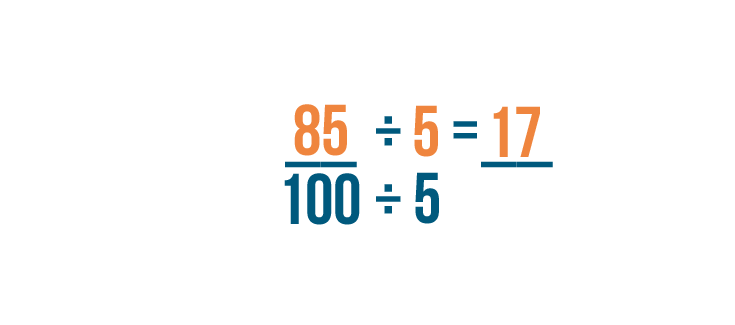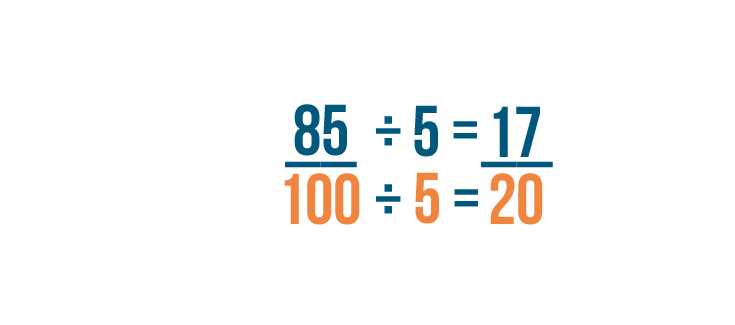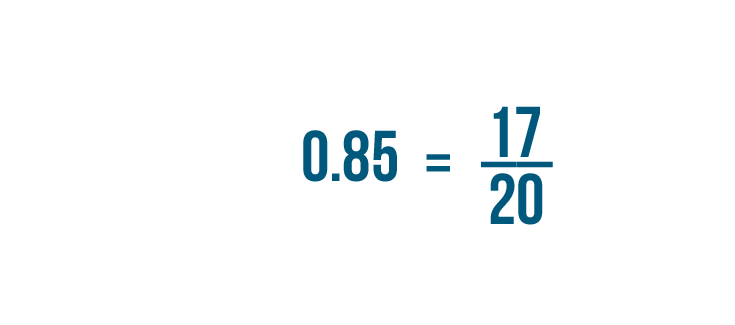Percents
Converting Percentages, Decimals, and Fractions
Converting fractions, decimals, and percents
When we talk, we often use different words to express the same thing. For example, we could describe the same car as tiny or little or small. All of these words mean the car is not big. Fractions, decimals, and percents are like the words tiny, little, and small. They're all just different ways of expressing parts of a whole.
In this image, each measuring cup has the same amount of juice in it. But we've expressed this amount in three ways: as a fraction, as a percent, and as a decimal. Since they're expressing the same amount, we know that 1/2, 50%, and 0.5 are equal to each other. Any time we see 1/2, we'll know it can also mean 50% or 0.5.
Sometimes it's useful to convert one kind of number into another. For example, it's much easier to add 1/4 and 0.5 if you turn 0.5 into a fraction. Learning how to convert fractions, decimals, and percents will also help you as you learn more advanced math.
Fractions and decimals
Every fraction can also be written as a decimal, and vice versa. You may not do this very often, but converting decimals and fractions can help you in math. For example, it's easier to subtract 1/6 from 0.52 if you turn 1/6 into a decimal first.
Converting a fraction into a decimal
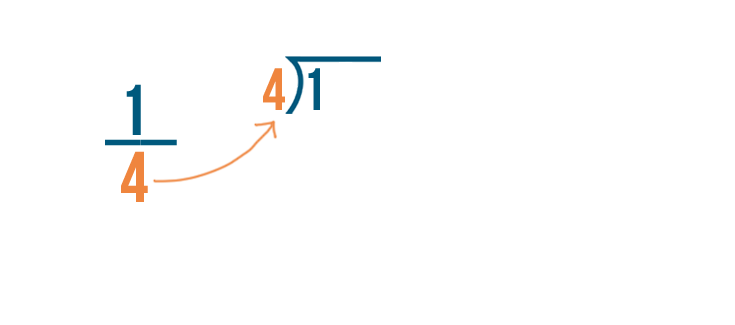
Let's convert a fraction into a decimal. We'll be using a math skill you've already learned: long division. To refresh your memory on this skill, you can review our Long Division lesson.
Click through the slideshow to learn how to convert a fraction into a decimal.
Let's see how we can convert 1/4 into a decimal.
To convert a fraction into a decimal, we'll just divide the numerator...
To convert a fraction to a decimal, we'll just divide the numerator...by the denominator.
In our example, we'll divide 1 by 4.
1 divided by 4 equals 0.
To keep dividing, we'll add a decimal point and a zero after the 1.
We'll also add a decimal point after the 0 on top.
Now we can divide 10 by 4.
10 divided by 4 equals 2.
Now we'll multiply 4 by 2.
4 times 2 equals 8. So we'll subtract 8 from 10.
10 minus 8 equals 2.
Since 2 is greater than 0, we're not finished dividing yet. We'll add another 0 after the decimal point and bring it down.
Now we'll divide 20 by 4.
20 divided by 4 equals 5.
Now we'll multiply. 4 times 5 equals 20.
When we subtract 20 from 20, we get 0. The 0 means we're done dividing.
1 divided by 4 equals 0.25.
So 1/4 is equal to 0.25.
Now it's your turn. Convert each of these fractions into a decimal.
Converting a decimal into a fraction
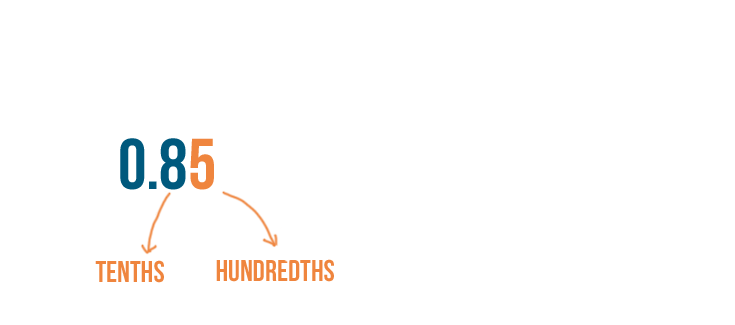
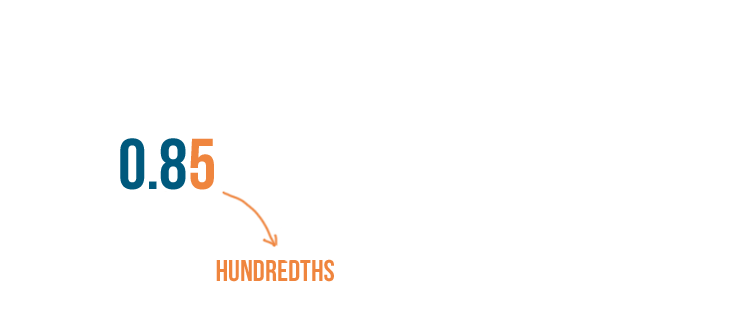
Now we'll do it in reverse. Let's convert a decimal into a fraction.
Click through the slideshow to see how to convert a decimal into a fraction.
We're going to rewrite 0.85 as a fraction. To convert a decimal into a fraction, we'll use place values.
In decimals, the number immediately to the right of the decimal point is in the tenths place.
The place to the right of the tenths place is the hundredths place.
To convert a decimal, first we'll check the place value of the last number to the right.
In 0.85, 5 is in the hundredths place.
This means our decimal is equal to 85 hundredths. 85 hundredths can also be written as 85/100.
Now we have our fraction. But it's always a good idea to reduce fractions when we can—it makes them easier to read.
To reduce, we need to find the largest number that will go evenly into both 85 and 100.
5 is the largest number that goes evenly into 85 and 100. So we'll divide both parts of our fraction by 5.
First we'll divide the numerator. 85 divided by 5 equals 17.
Now we'll divide the denominator. 100 divided by 5 equals 20. This means 85/100 can be reduced to 17/20.
So 0.85 is equal to 17/20.
Reducing a fraction may seem unnecessary when you're converting a decimal. But it's important if you're going to use the fraction in a math problem. If you're adding two fractions, you may even need to reduce or change both fractions so they have a common denominator.
Now you try it. Convert these decimals into fractions. Be sure to reduce each fraction to its simplest form!
