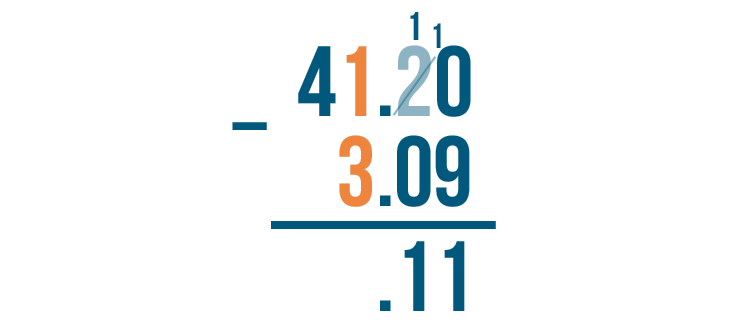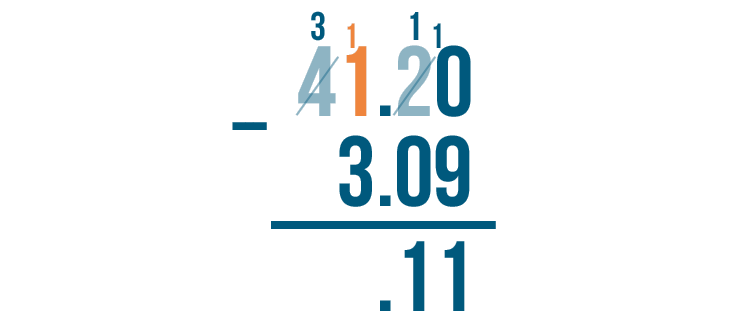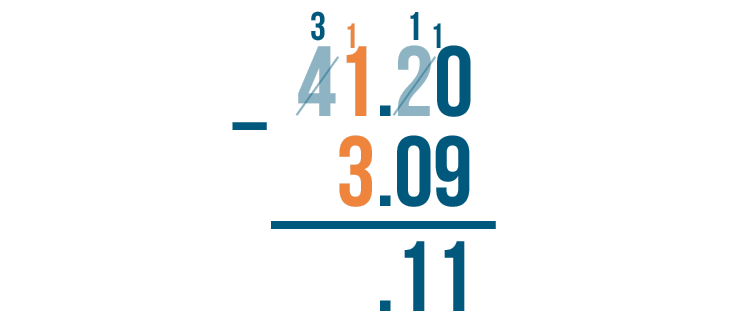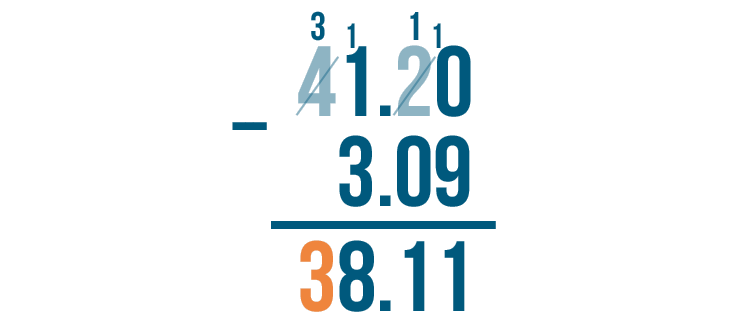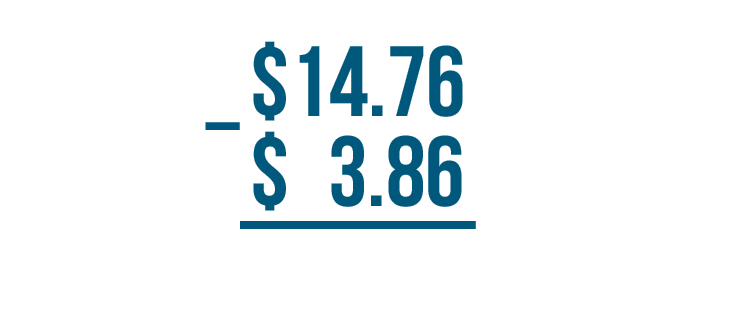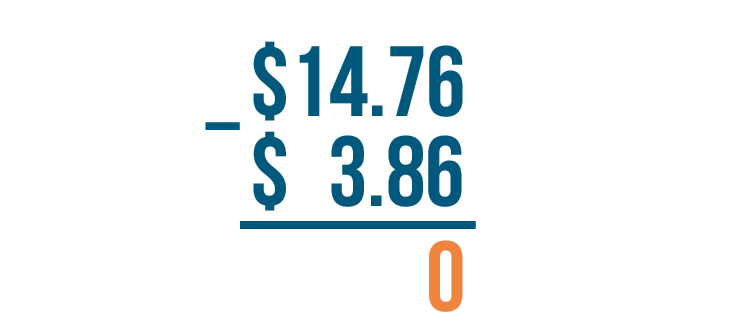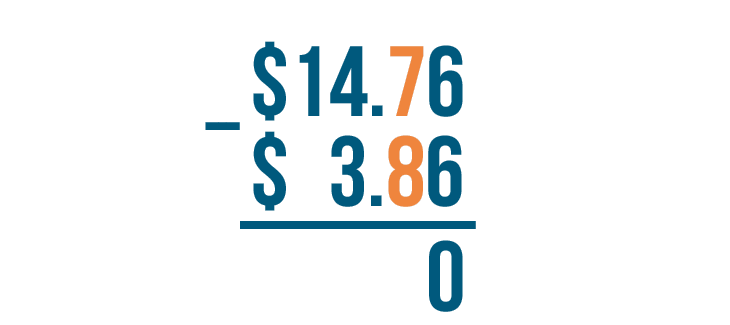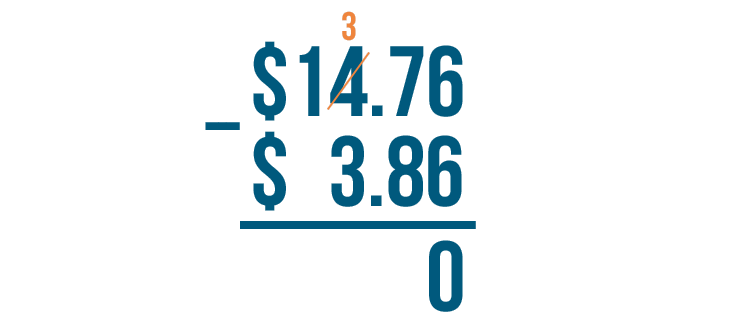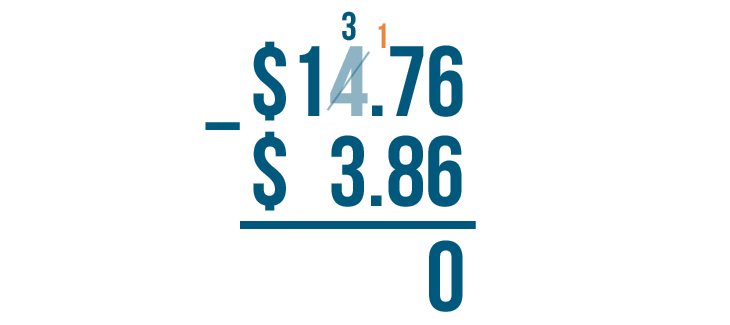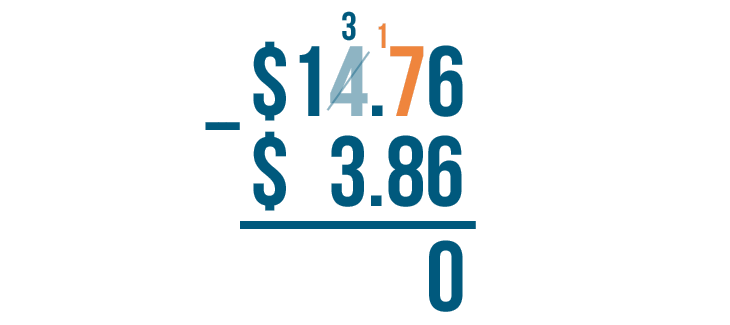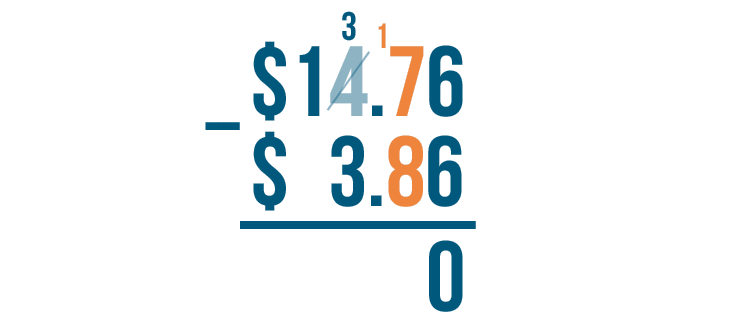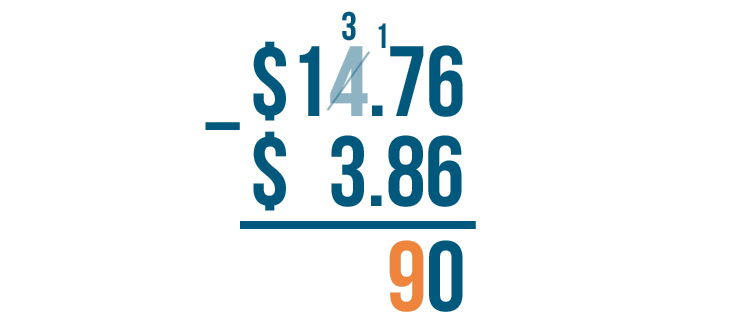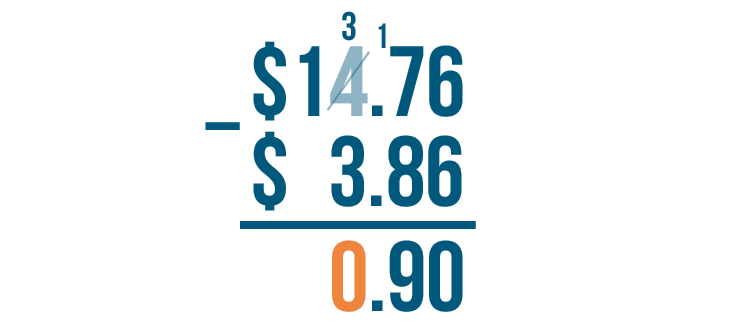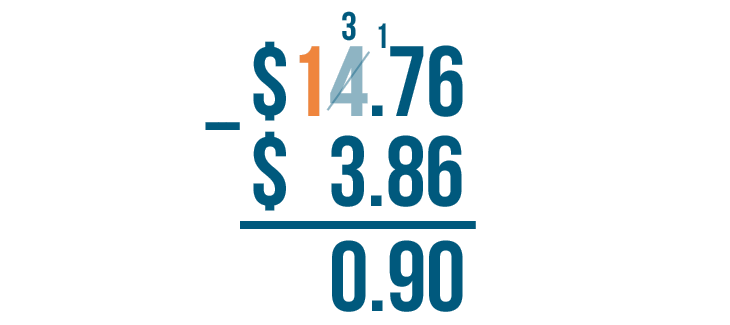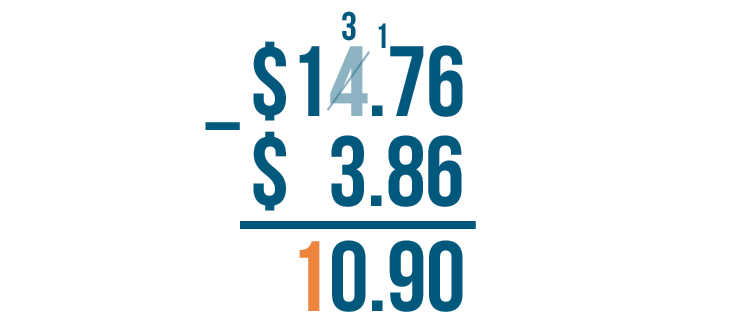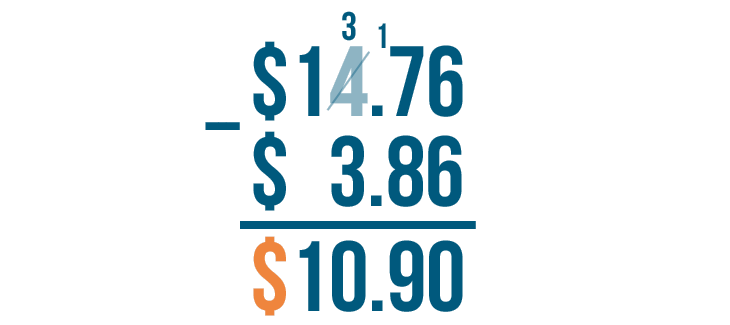Click through the slideshow to learn how to subtract decimals.

Let's try to solve this problem: 41.2 - 3.09.

First, we'll make sure the expression is set up correctly. Here, 41.2 is the larger number, so we'll put it on top.

The decimal points are lined up.

As always, we’ll begin with the digits farthest to the right. Here, we have nothing on top and 9 on the bottom.

We can’t take 9 away from nothing. We'll need to place a digit after 41.2 so we can subtract from it.

The value of our number won't change if we use the digit that means nothing: 0. We'll place a 0 after 41.2.

Now we can subtract the digits on the right. 0 is smaller than 9, so we’ll need to borrow to make 0 larger.

We learned how to borrow in the lesson on Subtracting Two- and Three-Digit Numbers.

We'll borrow from the digit to the left of 0. Here, it's 2. We'll take 1 from it.

2 - 1 = 1. To help us remember we subtracted 1, we'll cross out the 2 and write 1 above it.

Then we'll place the 1 we took next to the 0.

0 becomes 10.

10 is larger than 9, which means we can subtract. We'll solve for 10 - 9.
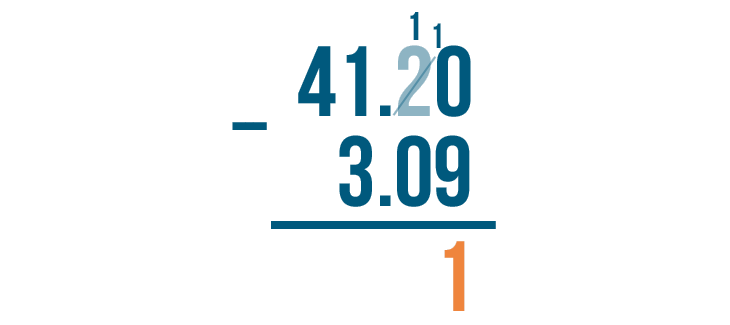
10 - 9 = 1. We'll write 1 beneath the line.

Now we'll move left to subtract the next set of digits: 1 - 0.
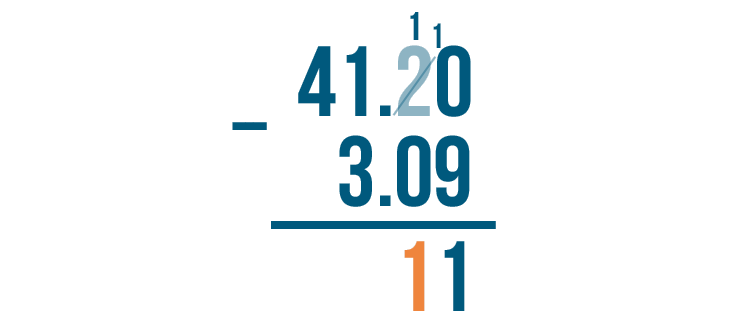
1 - 0 = 1. We'll write 1 beneath the line.
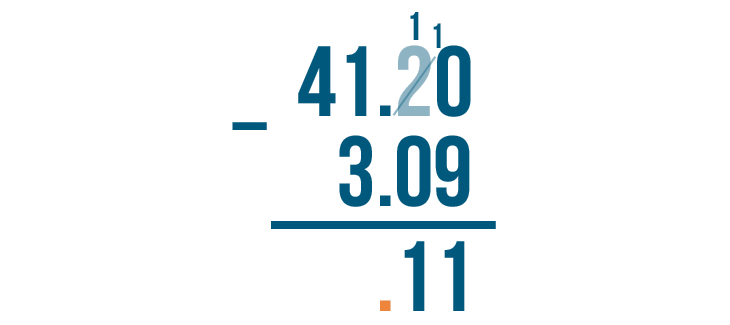
Now it's time to write the decimal point. We'll place it directly beneath the other two decimal points.
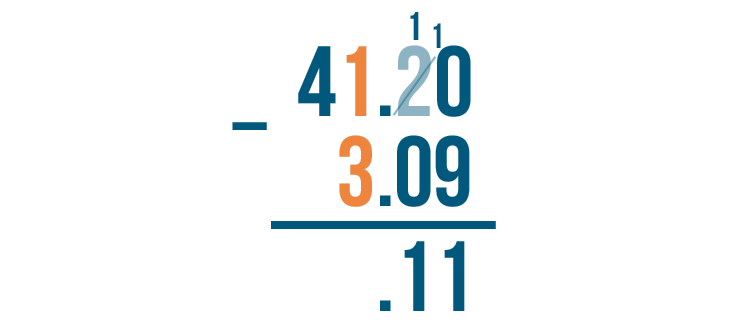
Now we'll find the difference of the next set of digits to the left: 1 - 3.
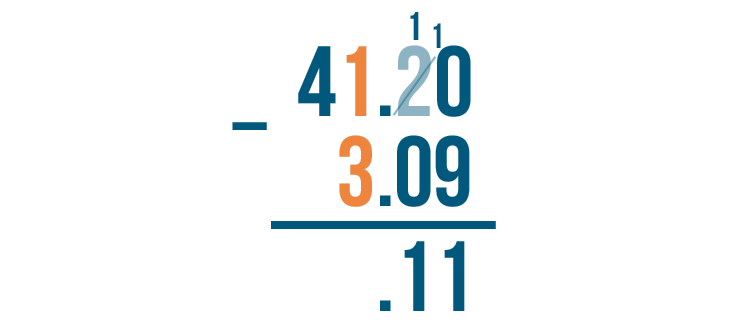
Because 1 is smaller than 3, it looks like we'll need to borrow again. We need to make the 1 larger.
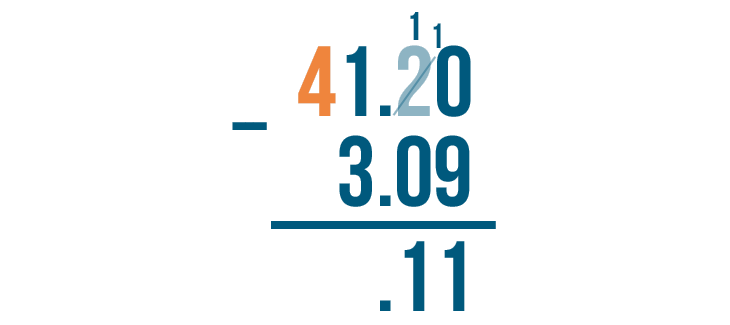
We'll borrow from the digit to the left of 1. Here, we'll borrow 1 from the 4.
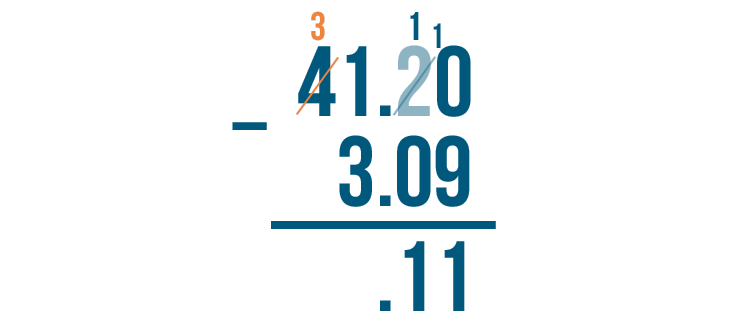
4 - 1 = 3. We'll write 3 above the 4.

Then we'll place the 1 we took next to the 1.
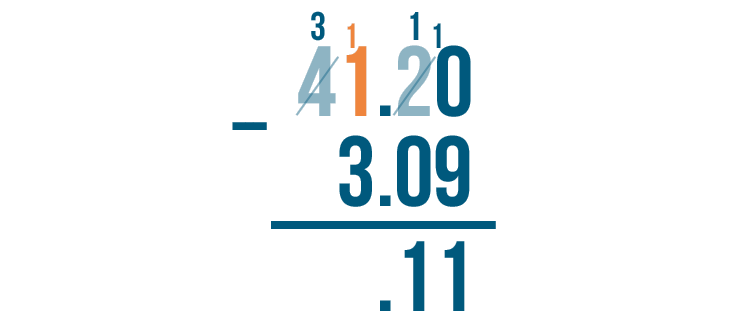
1 becomes 11.
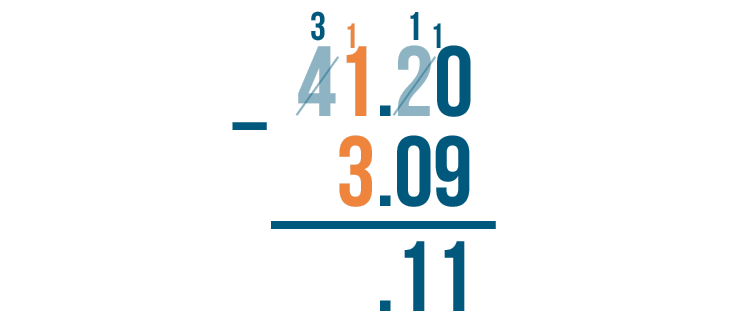
11 is larger than 3, which means we can subtract. We'll solve for 11 - 3.

11 - 3 = 8. We'll write 8 beneath the line.
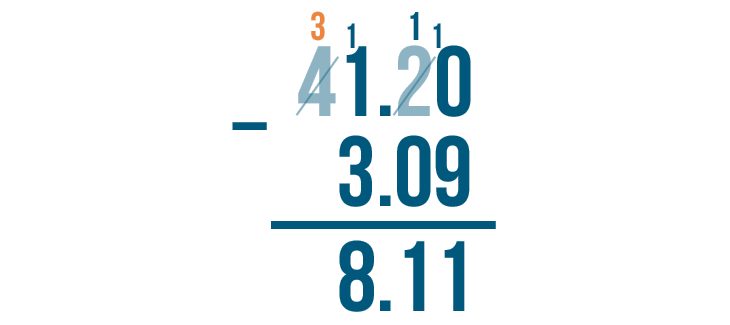
Finally, we'll move to the left to subtract the last set of digits. The top digit is 3, but there's nothing beneath it.
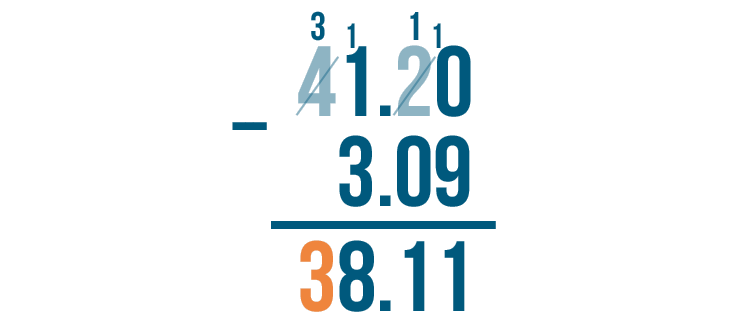
3 minus nothing equals 3, so we'll write 3 beneath the line.
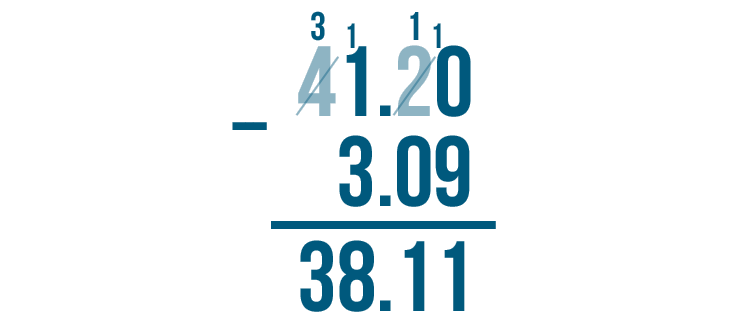
41.2 - 3.09 = 38.11. We can read this as thirty-eight and eleven-hundredths.
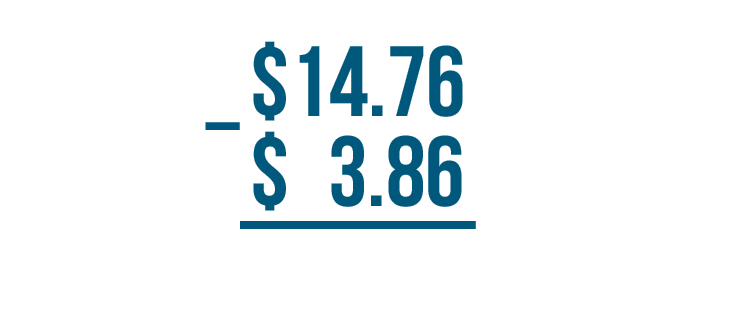
Let's try subtracting money. Let's see if we can solve $14.76 - $3.86.
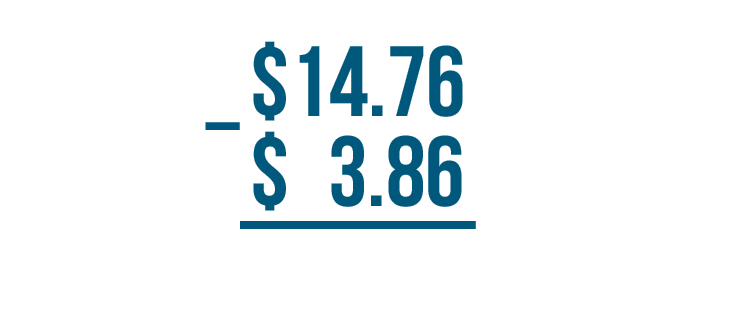
First, let's make sure the expression is set up correctly. The larger number is on top, and the decimal points are lined up.

As always, let's start by finding the difference of the digits on the right. Here, that's 6 - 6.
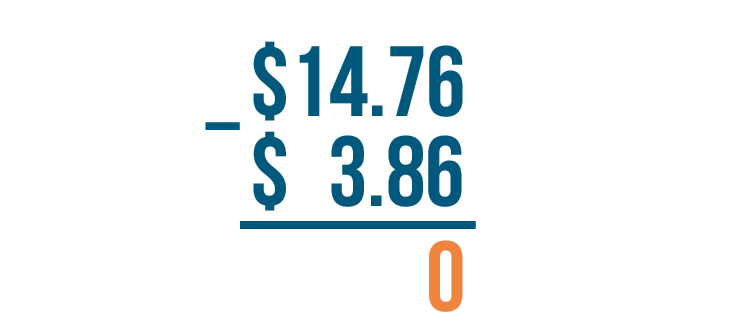
6 - 6 = 0. We'll write 0 beneath the line.
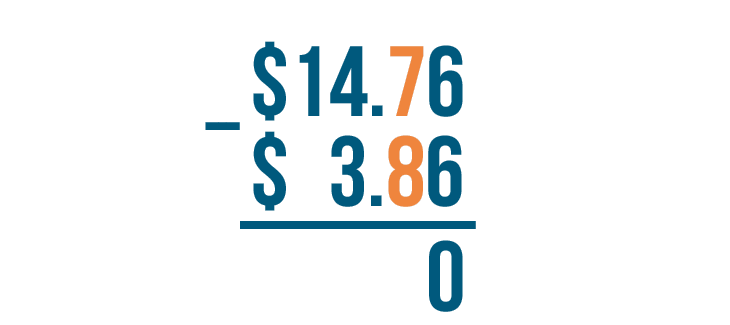
We'll move left to the next set of digits: 7 and 8. 7 is smaller than 8, so we'll borrow to make 7 larger.

Let's look at the digit to the left of 7. Here, it's 4. We'll take 1 from it.
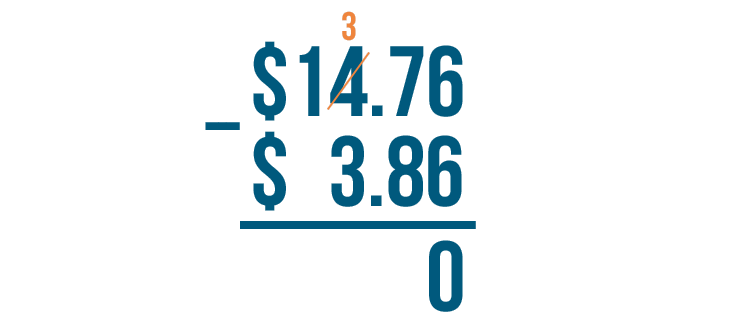
4 - 1 = 3. We'll cross out the 4 and write 3 above it.
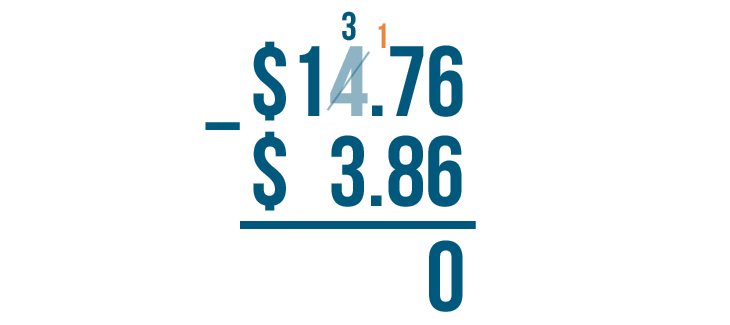
Then we'll place the 1 we took next to the 7.
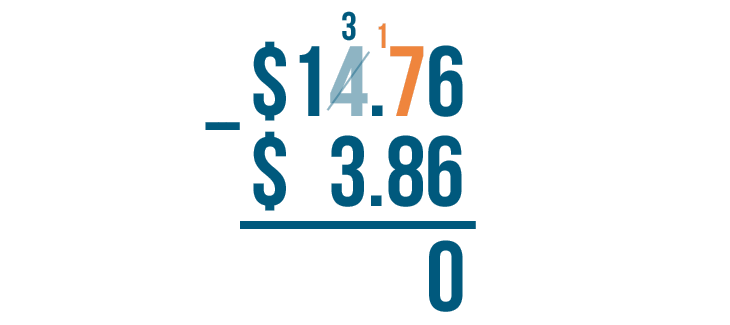
7 becomes 17.
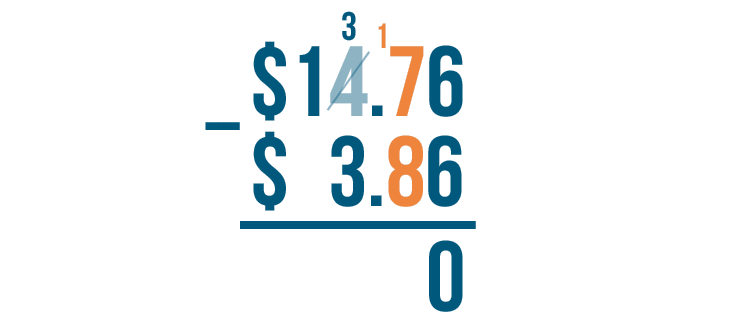
Now it's time to subtract. We'll solve for 17 - 8.
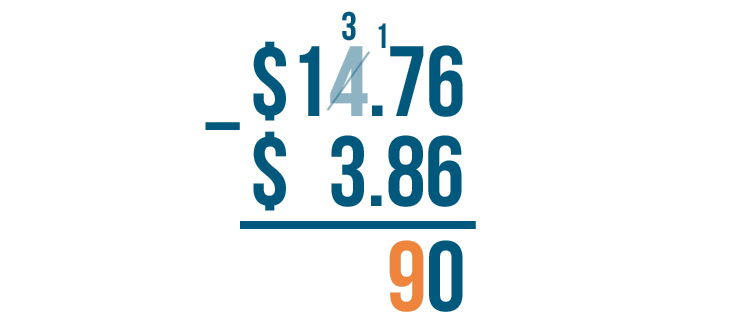
17 - 8 = 9. We'll write 9 beneath the line.

We'll put a decimal point directly beneath the other two decimal points.

Next, we'll move left to find the difference of the next set of digits. Here, that's 3 - 3.
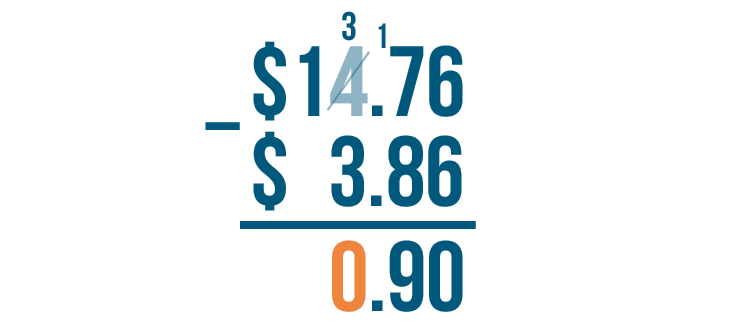
3 - 3 = 0. We'll write 0 below the line.
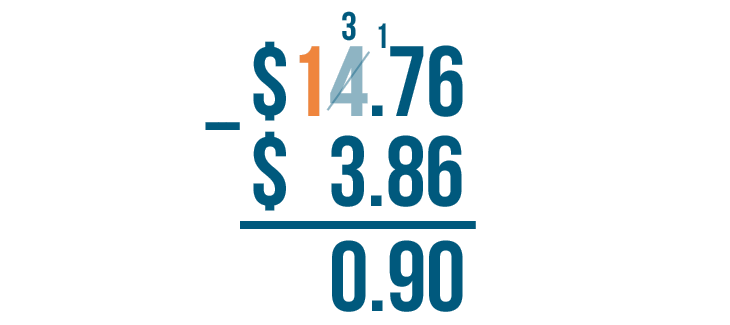
Finally, we'll move left to subtract the last set of digits. The top digit is 1, but there's nothing beneath it.
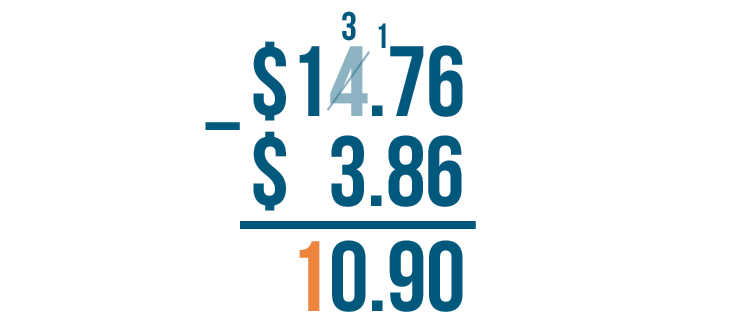
1 minus nothing equals 1. We'll write 1 beneath the line.
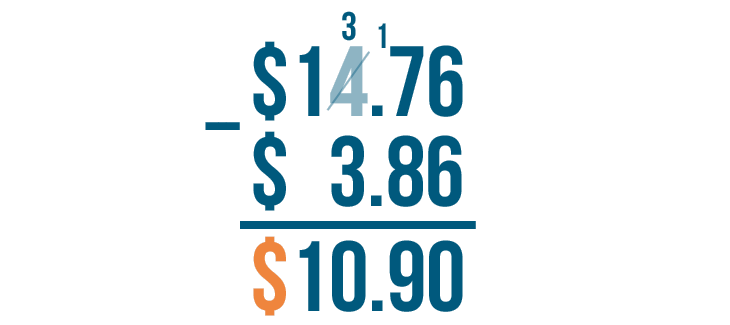
Next, we'll write a dollar sign ($) to the left of the 1.

$14.76 - $3.86 = $10.90. We can read this as ten dollars and ninety cents.