Practice Problems
Problems
In problems 1 – 3, use the shapes and slopes of the data to match the given numerical triples to the graphs in the figures. (For example, A: 3, 3, 6 in Problem 1. is "over and up" so it matches graph (a) in Fig. 14. B is "down and over" so it matches
graph (c) in Fig. 14.)
1. Fig. 14. Data: A: 3, 3, 6 B: 12, 6, 6 C: 7, 7, 3 D: 2, 4, 4

3. Fig. 16. Data: A: 7, 14, 10 B: 23, 45, 22 C: 0.8, 1.2, 0.8 D: 6, 9, 3

5. Water is flowing into each of the bottles in Fig. 18 at a steady rate. Match each bottle shape with the graph of the height of the water as a function of time.

(d) evaluate ,
and
9. Find the slope of the line through the points and
when
(c) ,
What are the values of these slopes in (
) if
,
,
?
11. and
. Evaluate and simplify
and
when
.
13. The graph in Fig. 21 shows the distance of an airplane from an airport during a several hour flight.

(a) How far was the airplane from the airport at 1 pm? At 2 pm?
(b) How fast was the distance changing at 1 pm?
(c) How could the distance from the plane to the airport remain unchanged from 1:45 pm until 2:30 pm without the airplane falling?
15.

a) Sketch the lines tangent to the curve in Fig. 23 at , and
.
b) For what value(s) of is the value of the function largest? Smallest?
c) For what value(s) of is the slope of the tangent line largest? Smallest?
17. Imagine that you are ice skating, from left to right, along the path in Fig. 25. Sketch the path you will follow if you fall at points , and
.

19. Let and define
to be the slope of the line through the points
and
in Fig. 27 . (For example,
= {slope of the line through
and
} =
).

a) Evaluate ,
and
.
b) Find an equation for in terms of
.
21. Use the graph of in Fig. 29 to complete the table. (You will have to estimate the values of the slopes).
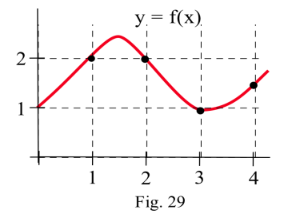
| slope of the line tangent to the graph of |
||
|---|---|---|
| 0 | 1 | 1 |
| 1 | ||
| 2 | ||
| 3 | ||
| 4 |
23. Design bottles whose graphs of (highest) water height versus time will look like those in Fig. 30.

Source: Dale Hoffman, https://s3.amazonaws.com/saylordotorg-resources/wwwresources/site/wp-content/uploads/2012/12/MA005-1.2-Lines-in-the-Plane.pdf
 This work is licensed under a Creative Commons Attribution 3.0 License.
This work is licensed under a Creative Commons Attribution 3.0 License.