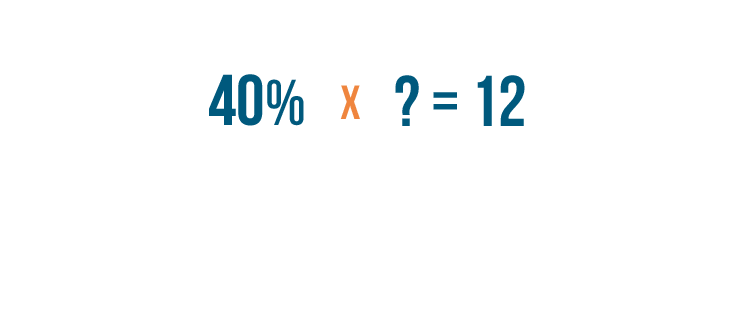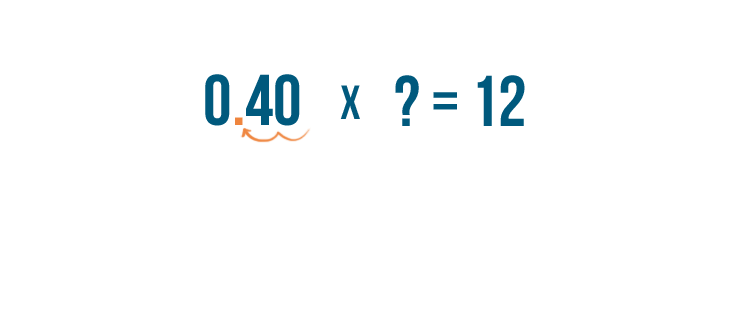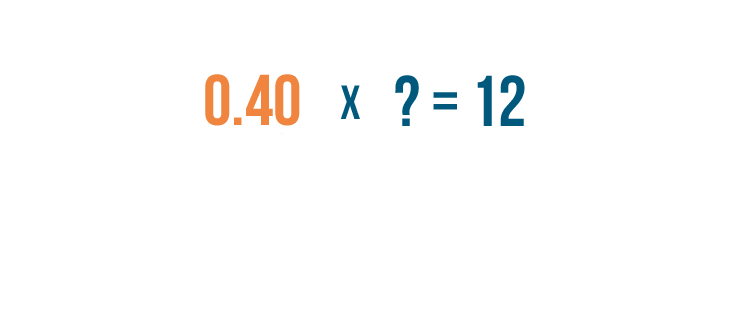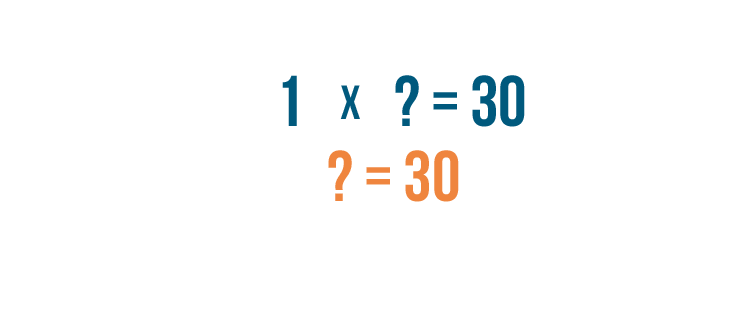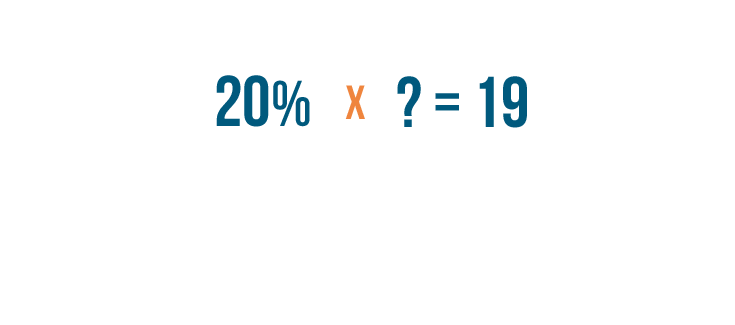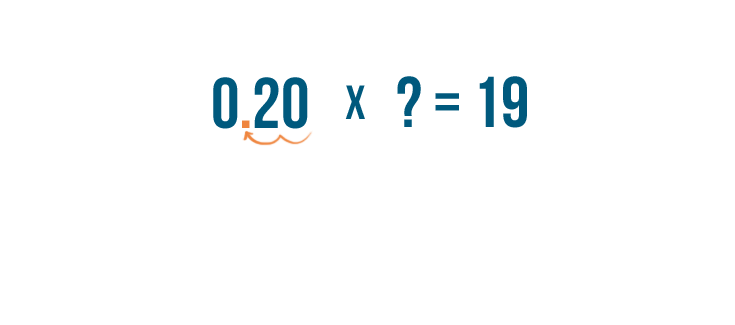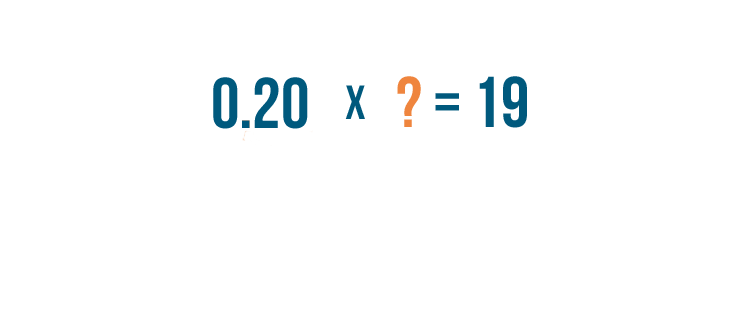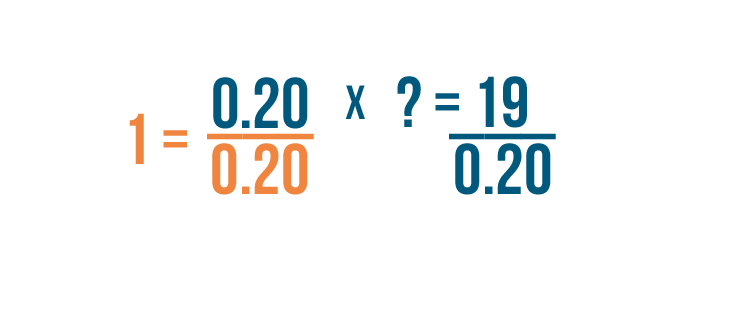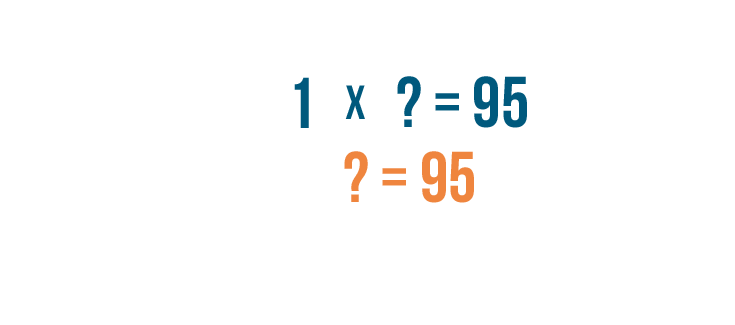Percents

Finding the total

Over the past week, 40% of the phone calls to Alicia's cell phone were from her son. Let's say you know that her son called her 12 times. Now you want to find out how many total calls she received. We can rewrite this example like this:

40% of ? = 12

This time we're missing the total—we don't know how many calls Alicia received.

Click through the slideshow to see how to find the total.

• 

  We know that 12 is 40% of our missing total. We want to find out what the total is.

• 

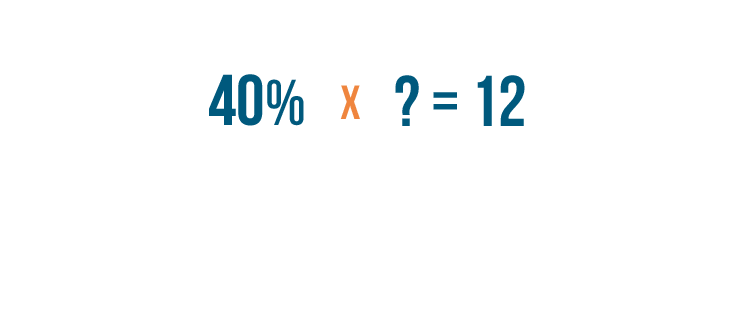
  First, we'll replace the word of with a multiplication sign.

• 

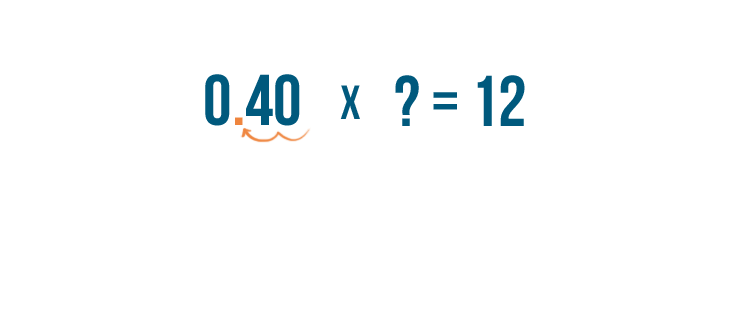
  Then we'll turn the percent into a decimal by moving the decimal point two spaces to the left. Now our problem is ready to solve.

• 

  First, we'll have to get the missing number alone on one side of the equals sign so we'll know what it's equal to.

• 

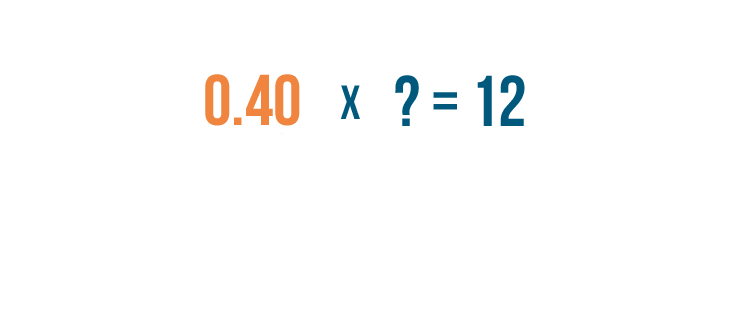
  To get the missing number alone, we'll need to get rid of the 0.40.

• 

  We'll divide 0.40 by itself to get rid of it.

• 

  The equals sign means the two sides of the expression are equal, so we have to divide the other side by 0.40 too.

• 

  Let's start with the left side of the equation. 0.40 divided by 0.40 is equal to 1.

• 

  We'll replace 0.40 with 1. 12 divided by 0.40 equals 30.

• 

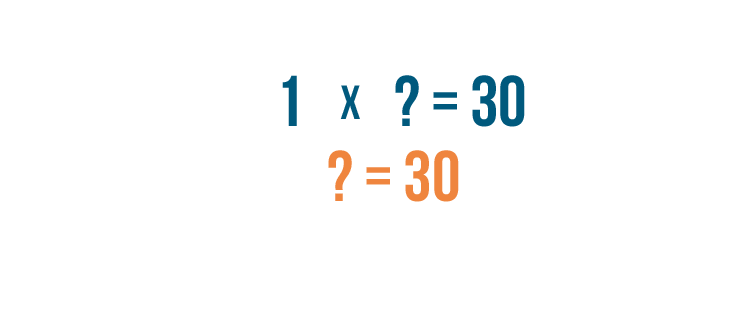
  Now we know that 1 times our missing number is equal to 30. So our missing number is also equal to 30.

• 

  We can replace the question mark in our original example with 30. Now we know that 12 is 40% of 30.

• 

  Let's try another example. 20% of a missing number is equal to 19. We want to find the missing number.
  

• 

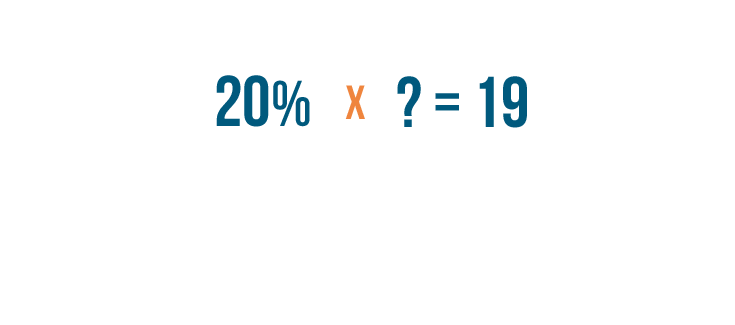
  Just like before, we'll replace the word of with a multiplication sign.

• 

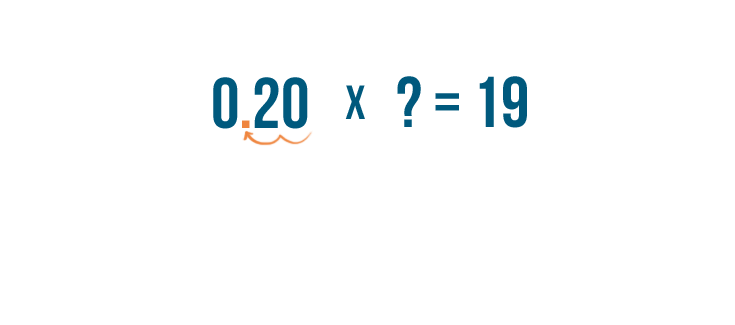
  Next, we'll turn our percent into a decimal by moving the decimal point two spaces to the left.

• 

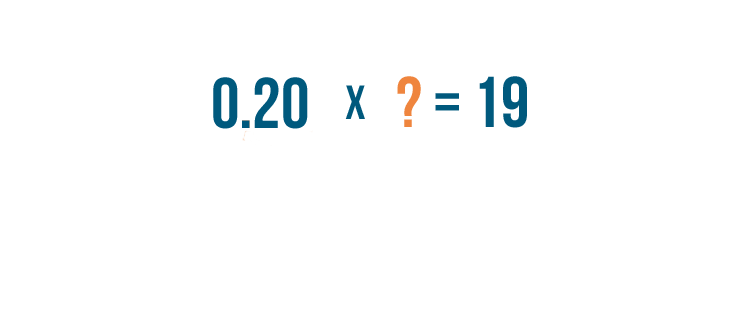
  Now we'll need to get our missing number alone on one side of the equals sign.

• 

  We'll divide 0.20 by itself to get rid of it. To keep the two sides equal, we'll have to divide the 19 by 0.20 too.

• 

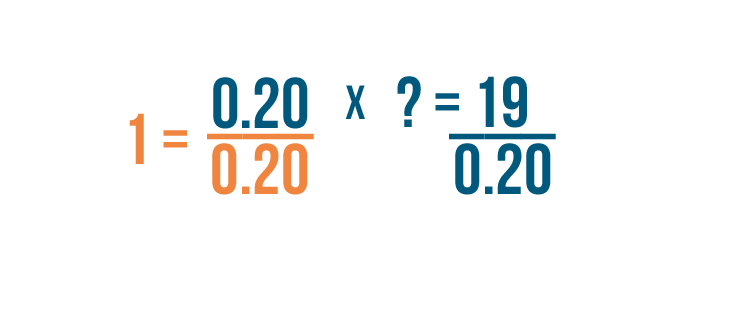
  0.20 divided by 0.20 equals 1.

• 

  We'll replace 0.20 with 1 and divide the other side of the equation. 19 divided by 0.20 equals 95.

• 

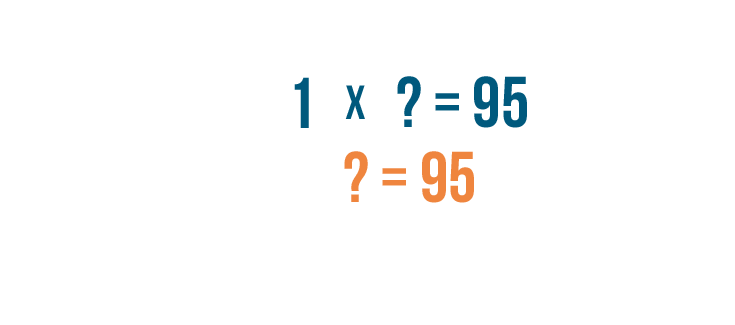
  Now we can see that 1 times our missing number equals 95. So our missing number is also equal to 95.

• 

  We can replace the question mark in our original example with 95. Now we know that 19 is 20% of 95.

• 

 Find the missing whole in each of these problems.

Four students are sharing an apartment. Each pays 25% of the rent. If each student pays $200 per month, how much is the total rent for each month?

Jill has a 25% off coupon for some computer software. If the coupon saved her $35, how much would the software cost without the coupon?

Allen paid $12 in interest on his credit card this month. If you know that the interest rate on his card is 6%, how much was the total amount on his card?