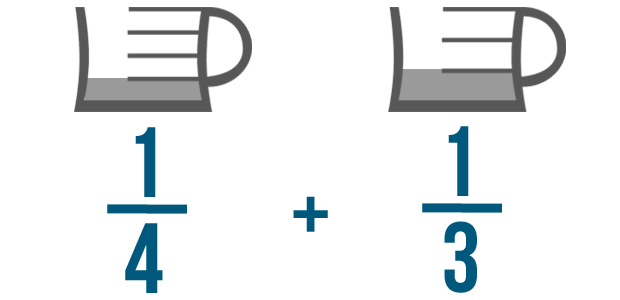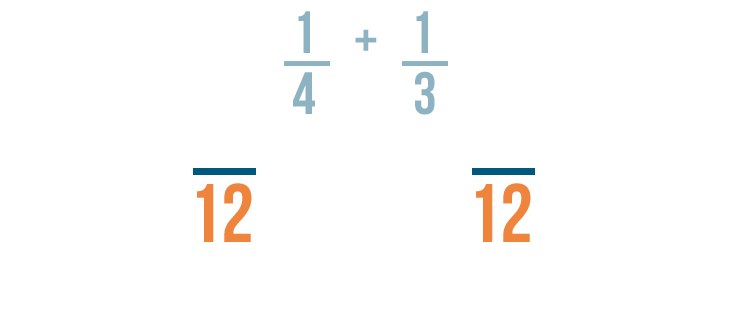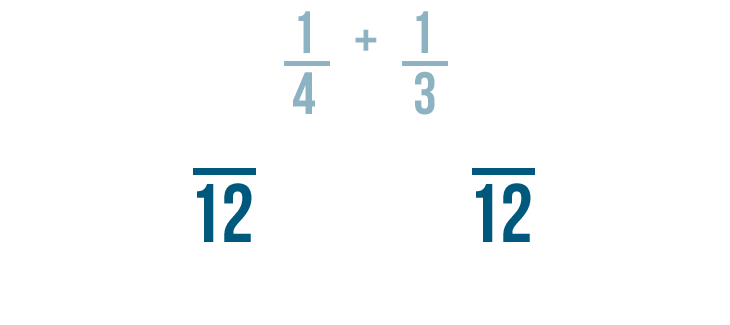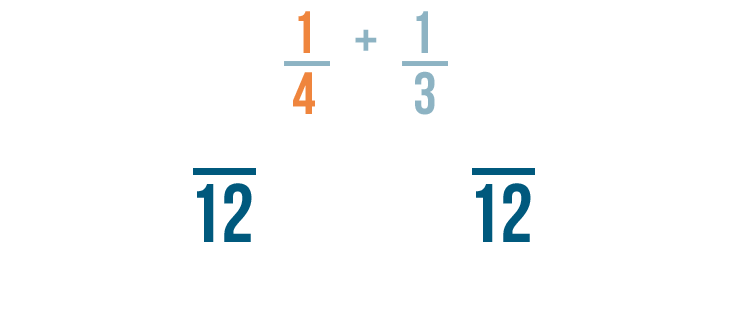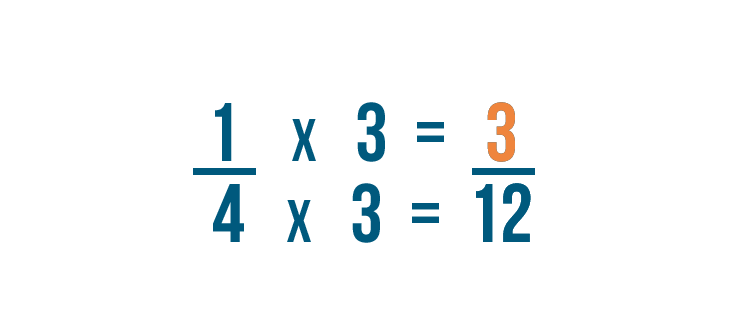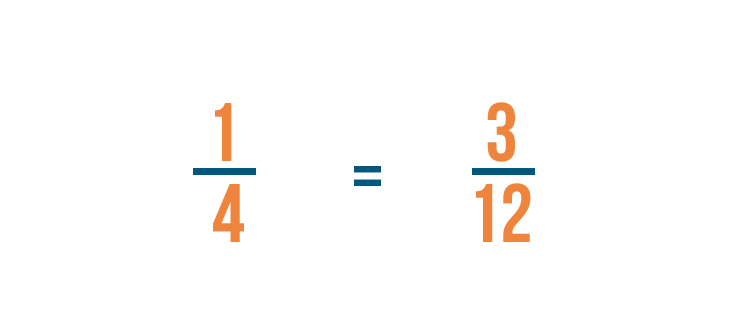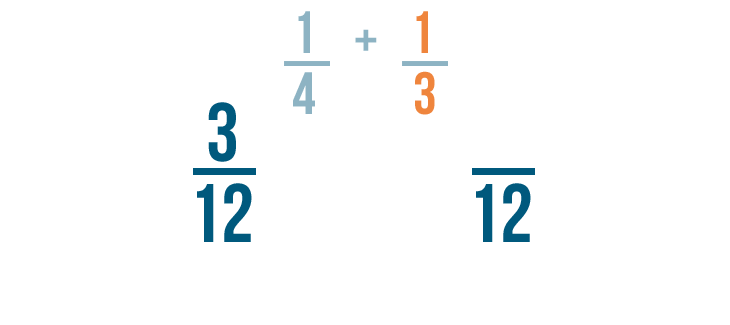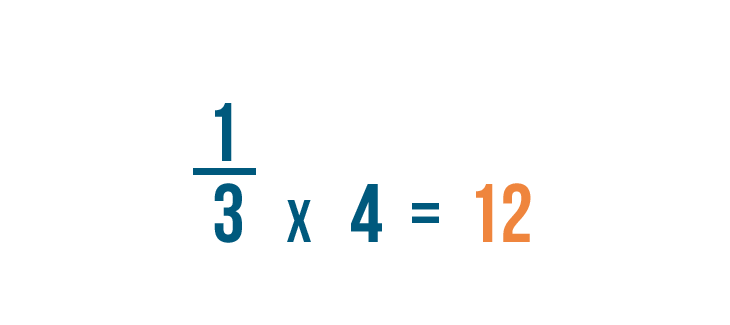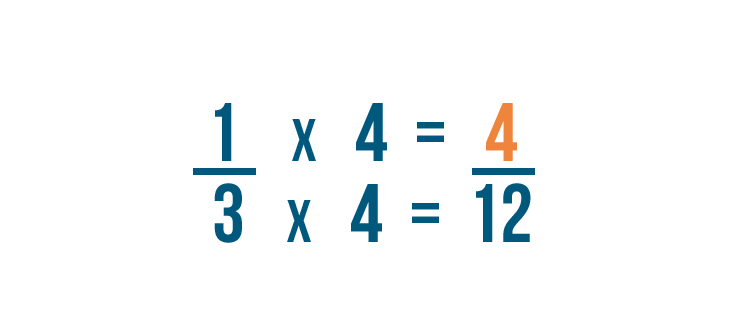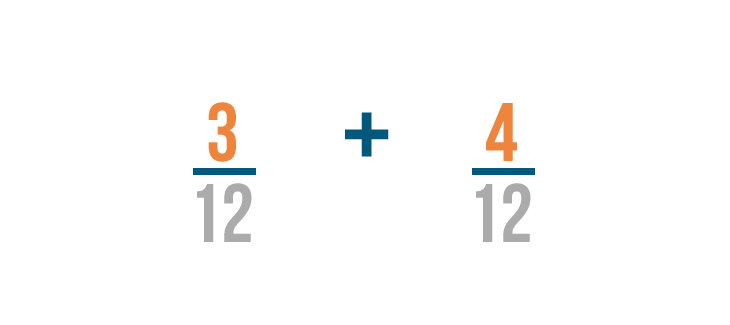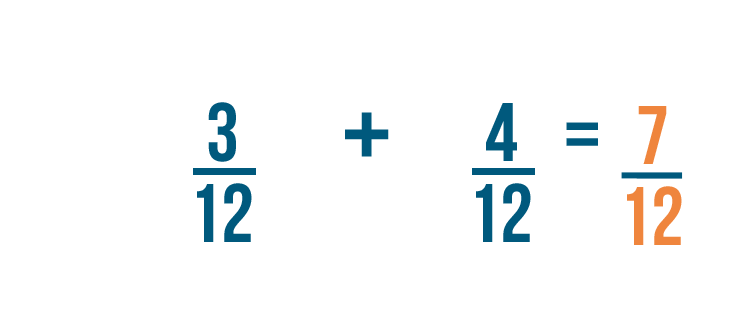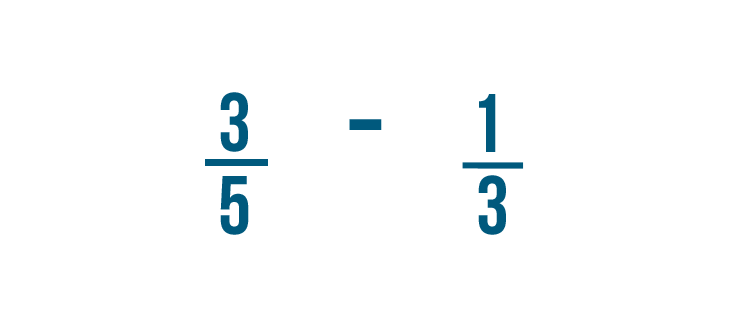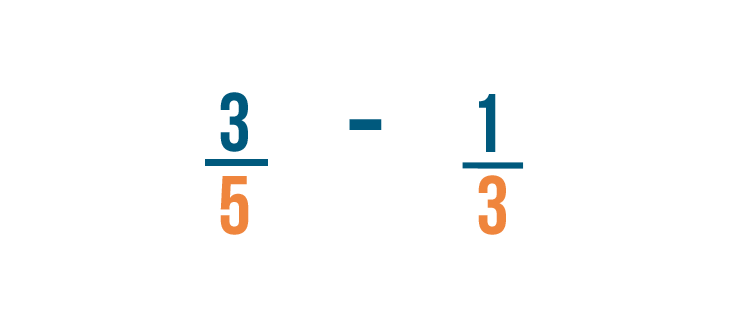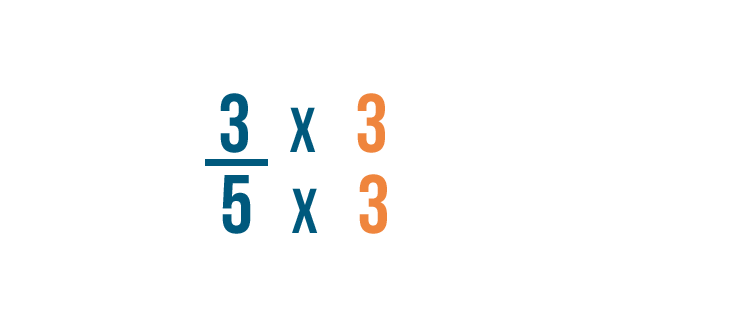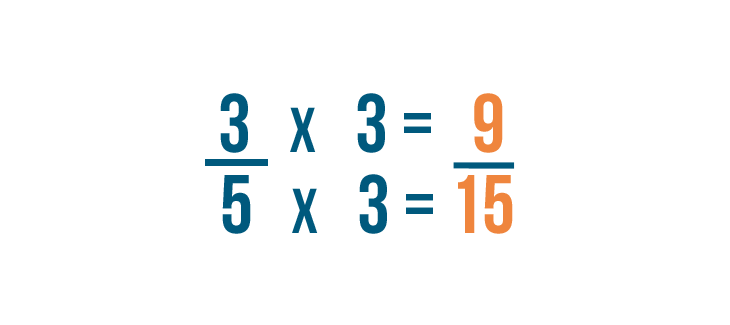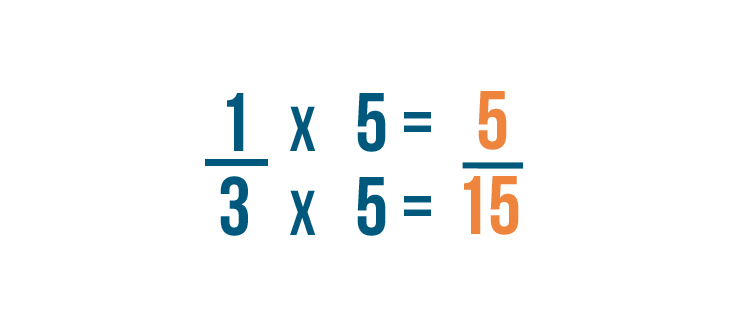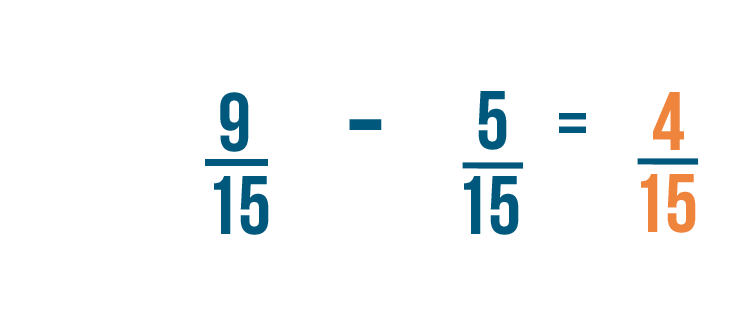Fractions

Adding fractions with different denominators

On the last page, we learned how to add fractions that have the same denominator, like 1/4 and 3/4. But what if you needed to add fractions with different denominators? For example, our cake recipe might say to blend 1/4 cup of milk in slowly and then dump in another 1/3 of a cup.

In Lesson 2, we compared fractions with a different bottom number, or denominator. We had to change the fractions so their denominators were the same. To do that, we found the lowest common denominator, or LCD. 

We can only add or subtract fractions if they have the same denominators. So we'll need to find the lowest common denominator before we add or subtract these fractions. Once the fractions have the same denominator, we can add or subtract as usual.

Click through the slideshow to learn how to add fractions with different denominators.

• 

  Let's add 1/4 and 1/3.

• 

  Before we can add these fractions, we'll need to change them so they have the same denominator.

• 

  To do that, we'll have to find the LCD, or lowest common denominator, of 4 and 3.

• 

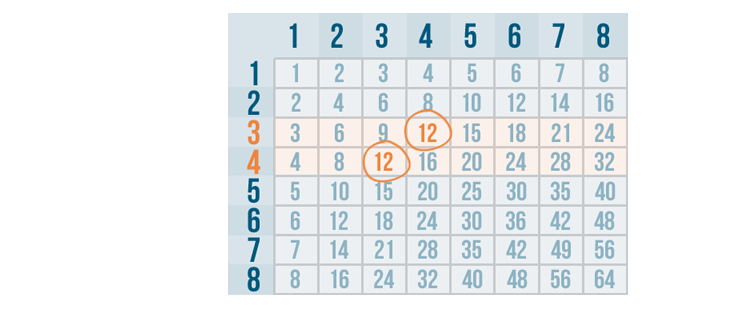
  It looks like 12 is the smallest number that can be divided by both 3 and 4, so 12 is our LCD.

• 

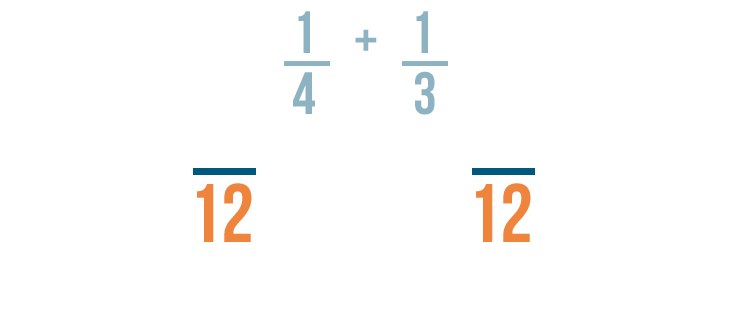
  Since 12 is the LCD, it will be the new denominator for our fractions.

• 

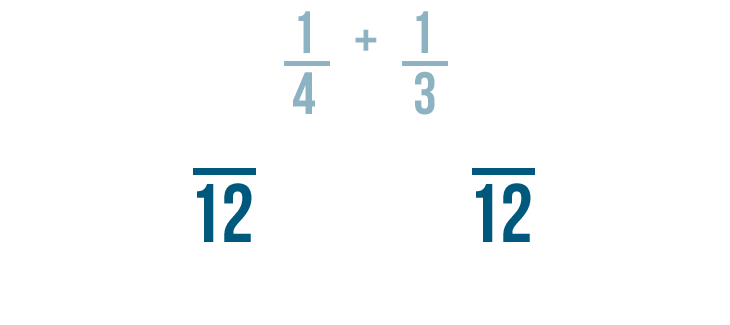
  Now we'll change the numerators of the fractions, just like we changed the denominators.

• 

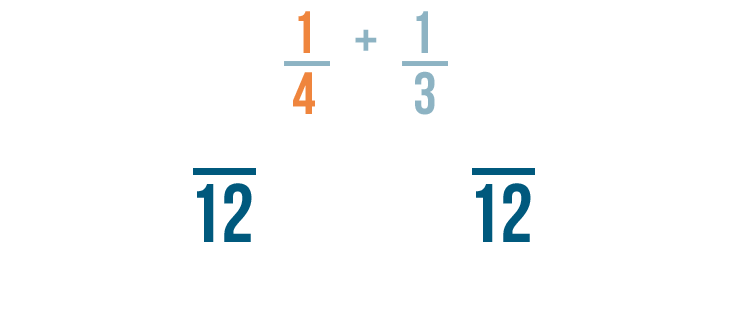
  First, let's look at the fraction on the left: 1/4.

• 

  To change 4 into 12, we multiplied it by 3.

• 

  Since the denominator was multiplied by 3, we'll also multiply the numerator by 3.

• 

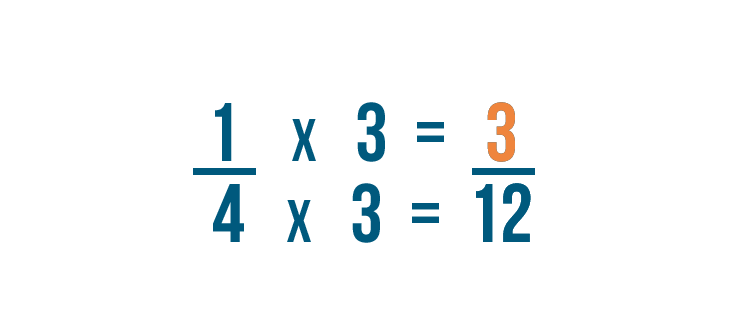
  1 times 3 equals 3.

• 

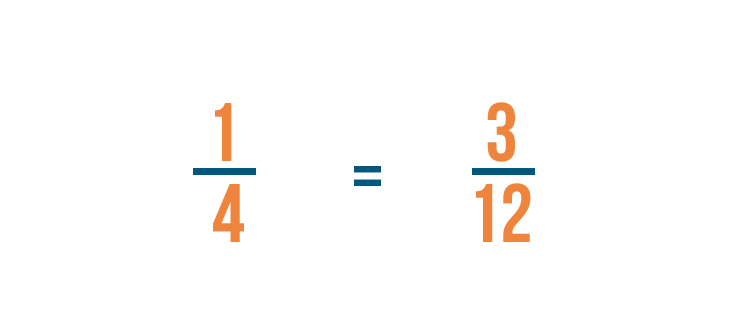
  1/4 is equal to 3/12.

• 

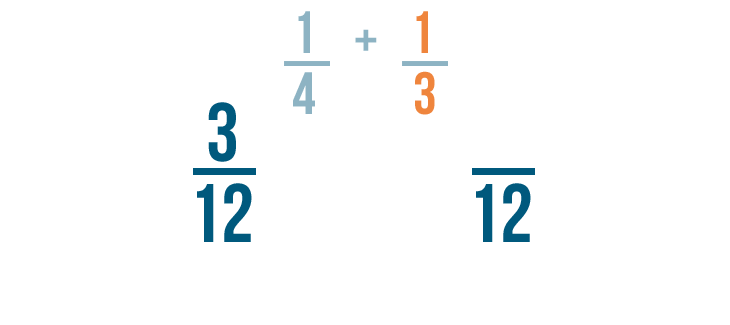
  Now let's look at the fraction on the right: 1/3. We changed its denominator to 12 as well.

• 

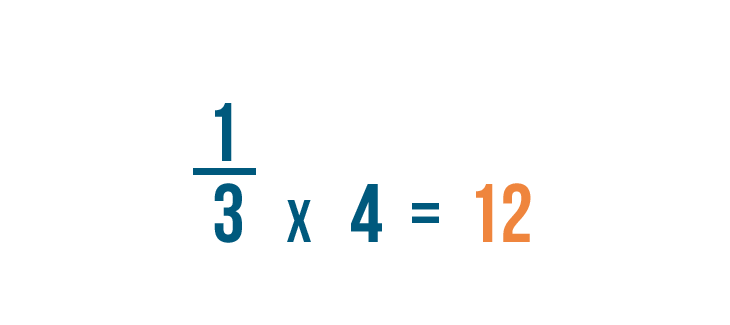
  Our old denominator was 3. We multiplied it by 4 to get 12.

• 

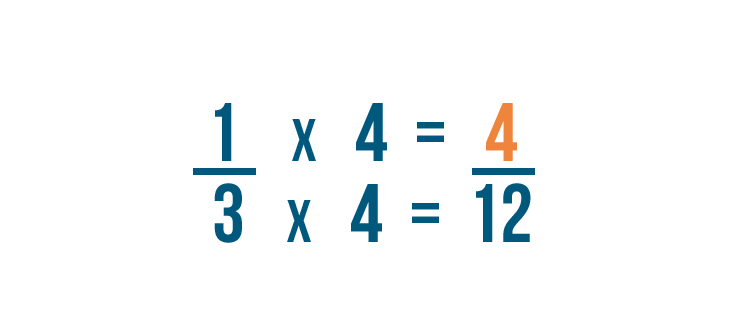
  We'll also multiply the numerator by 4. 1 times 4 equals 4.

• 

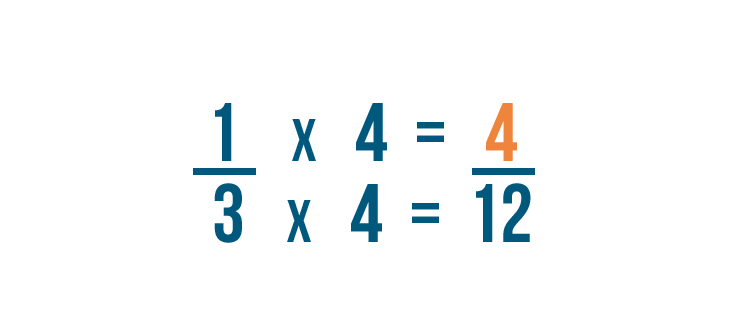
  So 1/3 is equal to 4/12.

• 

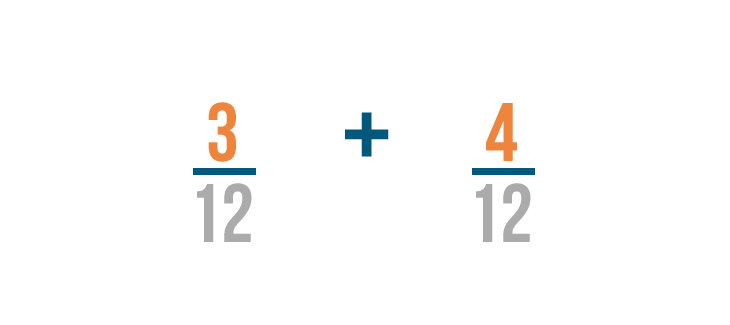
  Now that our fractions have the same denominator, we can add them like we normally do.

• 

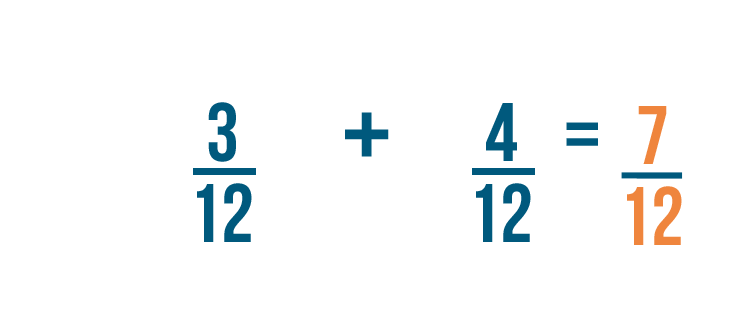
  3 plus 4 equals 7. As usual, the denominator stays the same. So 3/12 plus 4/12 equals 7/12.

• 

Now you try it! Try solving the addition problems below. Don't worry about reducing the answer for now.

Subtracting fractions with different denominators

We just saw that fractions can only be added when they have the same denominator. The same thing is true when we're subtracting fractions. Before we can subtract, we'll have to change our fractions so they have the same denominator.

Click through the slideshow to learn how to subtract fractions with different denominators.

• 

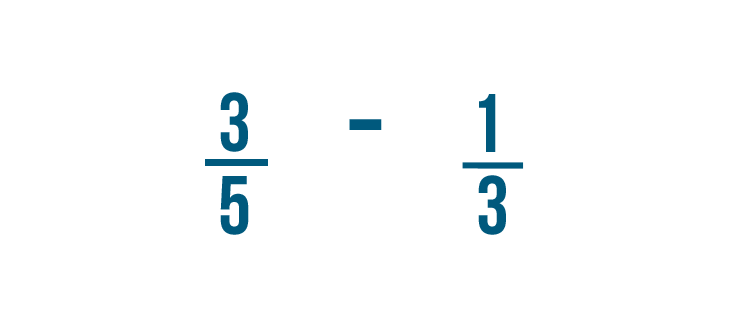
  Let's try subtracting 1/3 from 3/5.

• 

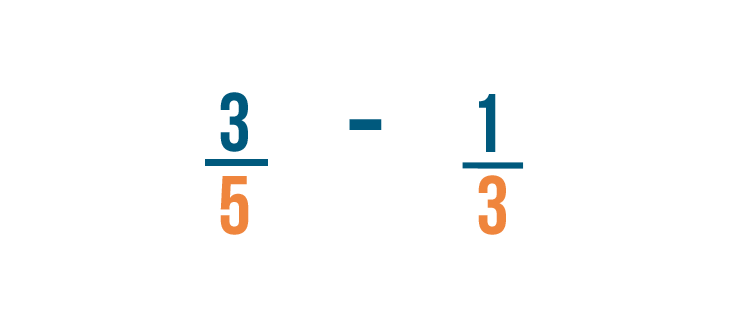
  First, we'll change the denominators of both fractions to be the same by finding the lowest common denominator.

• 

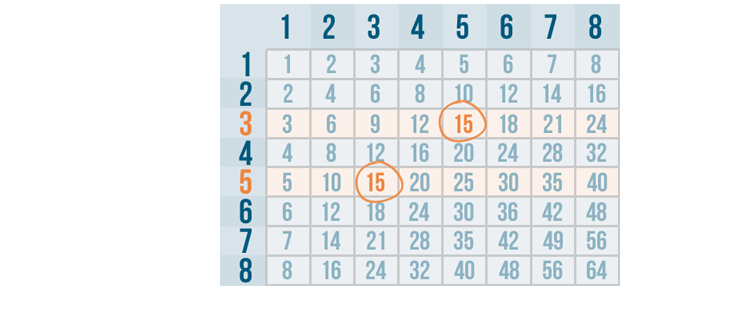
  It looks like 15 is the smallest number that can be divided evenly by 3 and 5, so 15 is our LCD.

• 

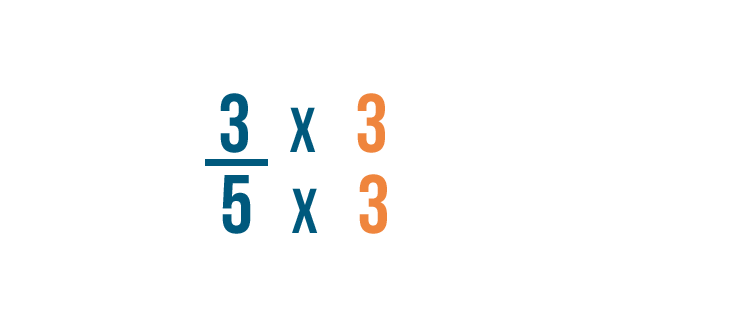
  Now we'll change our first fraction. To change the denominator to 15, we'll multiply the denominator and the numerator by 3.
  
  

• 

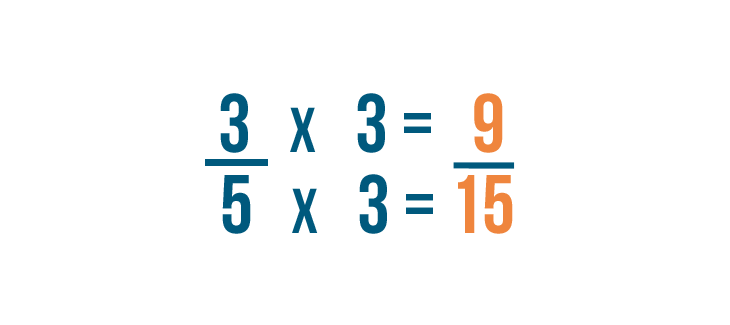
  5 times 3 equals 15. So our fraction is now 9/15.

• 

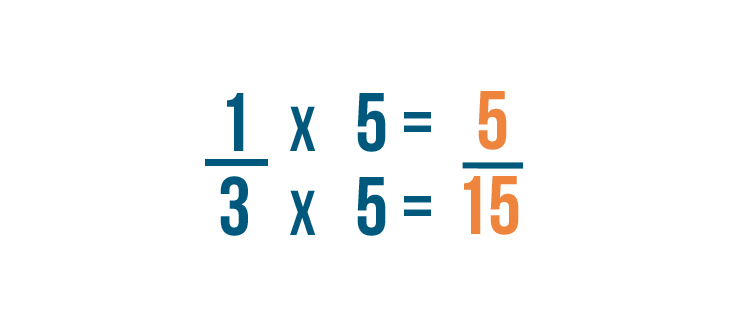
  Now let's change the second fraction. To change the denominator to 15, we'll multiply both numbers by 5 to get 5/15.
  
  

• 

  Now that our fractions have the same denominator, we can subtract like we normally do.

• 

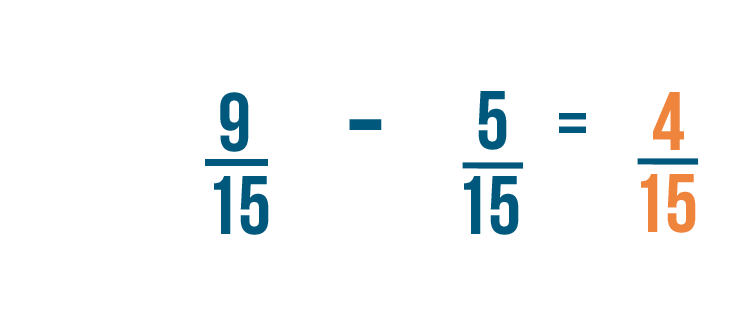
  9 minus 5 equals 4. As always, the denominator stays the same. So 9/15 minus 5/15 equals 4/15.

• 

Now you try it! Try solving the subtraction problems below. Don't worry about reducing the answer for now.